Overview
AS 4100:2020 Steel Structures uses the limit state method for the design of structural steel members. This method involves calculating factored loads and reduced capacities to account for variability in loading conditions and material properties. For the ultimate limit state (ULS) design to be satisfied, the following relationship must be true:
\(ULS \;Factor * Load ≤ Reduction \;Factor * Capacity\)
This document outlines the procedure for designing a steel member in accordance with AS 4100 using the SkyCiv AS 4100:2020 Steel Member Design module.
Contents
Material Properties
Fabrication
In Australia there are three typical forms of structural steel fabrication:
- Hot Rolled Sections: Steel billet (blocks) are heated, rolled through a mill into the desired shape, then cooled. Examples include Universal Beams/Columns, Parallel Flange Channels (PFC) and Angle sections.
- Cold Formed Sections: Steel billet is pressed at room temperature into the desired shape. Examples include Rectangular Hollow Sections (RHS) and Circular Hollow Sections (CHS).
- Fabricated Sections: Multiple hot rolled flat plates are welded together to form a steel section. Examples include Welded Beams/Columns.
Steel Grade
Australia has numerous steel grades (strengths) that can be used for design in accordance with AS 4100:2020. Grade availabilities for different section types are outlined below.
| Grade | WB / WC | UB / UC | PFC | EA / UA | RHS / SHS | CHS |
|---|---|---|---|---|---|---|
| 250 | NO | NO | NO | NO | NO | YES |
| 300 | YES | YES | YES | YES | NO | NO |
| 350 | YES | YES | YES | YES | YES | YES |
| 400 | YES | NO | NO | NO | NO | NO |
| 450 | NO | NO | NO | NO | YES | NO |
Yield Strength
The yield strength of a steel section is dependent on its Grade, with higher grades having higher yield stresses. The yield strength of Hot Rolled and Fabricated sections vary depending on the thickness of the section. Thicker steel sections typically have lower yield strengths than thinner sections of the same Grade.
Cold Formed Sections are the exception to this rule and have a consistent yield strength for each Steel Grade, regardless of section thickness. Section yield strength and ultimate (tensile) strength values can be calculated using Table 2.1 in AS 4100:2020.
Selecting a Section in SkyCiv AS 4100 Steel Member Design
The SkyCiv AS 4100:2020 Steel Member Design tool allows users to select a Standard section from the SkyCiv database or design a completely Custom section. When a Standard section is selected, flange and web yield strengths are automatically calculated based on the steel grade using AS 4100 Table 2.1. When a Custom section is selected, the user must input values for flange and web yield strength. Standard sections can also be used with a Custom steel grade if specified by the user.

Section Capacity
Bending
Section Bending Moment Capacity
AS 4100:2020 calculates the bending moment capacity of a steel section as follows:
\(M_s = f_y*Z_e\)
Where fy is the yield stress of the material, and Ze is the effective section modulus. The section modulus of a shape is a geometric property which quantifies the bending resistance of a shape. In structural engineering we use two section modulus values, the elastic (Z) and plastic (S) section modulus. Note, design standards in other regions sometimes interchange the symbols for elastic and plastic section modulus.
The elastic section modulus assumes the entire section (shape) remains elastic under bending, i.e. no part of the section exceeds the yield strength (fy) of the material. This generally occurs when the extreme fibers in the section (top/btm) reach yielding. The elastic section modulus of a section is calculated as follows:
\(Z = \frac{I}{y}\)
Where I is the second moment of area and y is the geometric centroid of the shape.
The plastic section modulus assumes that the entire section reaches the yield strength of the material under bending, which means parts of the section will exceed the yield strength and experience plastic deformation. The plastic section modulus of a section is calculated as follows:
\(S = A_C*y_C + A_T*y_T \)
Where AC and AT are the areas on either side of the Plastic Neutral Axis (PNA), and yc / yt are the distance from the PNA to the centroid of those areas. Note, the PNA location is equal to the geometric centroid location for symmetric shapes but will not equal the geometric centroid location for asymmetric shapes.
Section Classification
Some steel shapes may have elements of the shape locally buckle before reaching their yield strength, meaning the full capacity of the elastic/plastic section modulus cannot be achieved. This typically occurs in larger, thinner shapes, which are more susceptible to local buckling. AS 4100 uses the effective section modulus (Ze) value to account for the possibility of local buckling and reduce the bending capacity of the section accordingly. AS 4100 classifies sections into three categories:
- Compact: Compact sections are not susceptible to local buckling and can achieve their full plastic moment capacity, meaning the entire section can reach its yield strength under bending.
- Non-Compact: Non-compact sections can reach yield strength in the extreme fibres of the section (elastic moment capacity) but cannot achieve their plastic moment capacity before local buckling occurs.
- Slender: Slender sections cannot achieve their elastic moment capacity before local buckling occurs.

Section Slenderness
AS 4100 determines the section classification by calculating the slenderness of each element within a section and finding the “critical element” that will buckle in compression first. For an I-section, the elements are broken down as shown below. Slenderness values are only calculated for outstand elements, i.e. elements which are not restrained in both directions. The area of connection between a flange and web (shown in white below) is restrained in both directions and hence is not susceptible to local buckling.
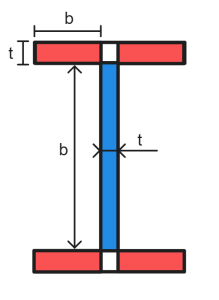
The slenderness of a flat element is calculated as follows:
\(λ_e = \frac{b}{t}\sqrt{\frac{f_y}{250}}\)
AS 4100 Table 5.2 contains values for plasticity and yield slenderness limits (λep & λey) for compression plate elements based on stress distribution, edge support and residual stresses. The critical element of a section is the element with the highest λe / λey ratio. The slenderness values of this element (λe) are used to classify the entire section (referred to as λs).
If λs ≤ λsp the section is compact. For compact sections, the effective section modulus is calculated as follows:
\(Z_e = Z_c = min(S,1.5*Z)\)
Where S is the plastic section modulus, and Z is the elastic section modulus of the section. The term Zc is used interchangeably for the effective section modulus of a compact section.
If λsp ≤ λs ≤ λsy the section is non-compact. For non-compact sections, the effective section modulus is calculated as follows:
\(Z_e = Z + [(\frac{λ_{sy} – λ_{s}}{λ_{sy} – λ_{sp}})(Z_c-Z)]\)
Where Zc is the effective section modulus for a compact section.
If λs > λsy the section is slender. For a slender section with flat plate elements in uniform compression, the effective section modulus is calculated as follows:
\(Z_e = Z(\frac{λ_{sy}}{λ_s})\)
Note, the effective section modulus for circular hollow sections or flat plat elements with tension at the unsupported edge is calculated differently. Refer AS 4100 Clause 5.2.5 for further information.
Calculating Section Bending Capacity in SkyCiv AS 4100 Steel Member Design
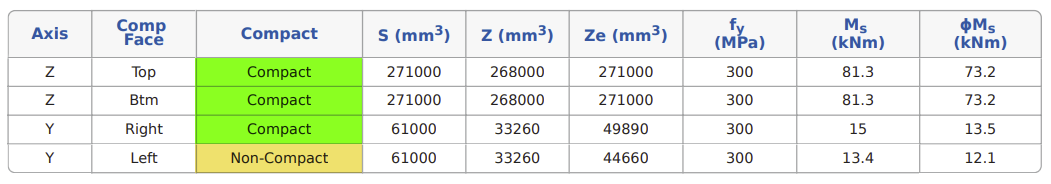
The SkyCiv AS 4100:2020 Steel Member Design tool calculates slenderness classifications and section bending capacities for positive and negative bending about both principal axes. The results of the slenderness classification check for a 230 PFC are detailed below.

It’s apparent that the slenderness values and section classification are different depending on the direction of bending. This is because the stress distributions and edge support values change depending on which elements are in compression or tension, resulting in different slenderness limit values.
Once section slenderness is known, the module calculates the section bending moment capacity (Ms) about each principal axis for positive and negative bending. For symmetric shapes (such as I-Sections), this value will be the same in the positive and negative direction. Asymmetric shapes will have different section bending capacities in the positive and negative bending direction, such as the 230 PFC shown in the example below.
Shear
Section Shear Capacity
AS 4100 only considers the web of a section to contribute to its shear capacity. Hence the shear capacity of a section (Vv) is equal to the web shear capacity. Vertical stiffeners can be added to a section to increase its shear capacity if required. The capacity of an unstiffened web is calculated differently depending on whether the shear stress distribution across the web is uniform or non-uniform. The following shear stress distributions are assumed for standard section shapes:
| Shape | Shear Stress Distribution |
|---|---|
| I-Section | Uniform |
| T-Section | Non-Uniform |
| Parallel Flange Channel (PFC) | Uniform |
| Rectangular Hollow Section (RHS) | Non-Uniform |
| Circular Hollow Section (CHS) | Uniform |
Uniform Shear Stress Distribution
The shear capacity of a section with uniform shear stress distribution (Vu) is calculated differently depending on the slenderness of the web panel. For a non-slender web, capacity is calculated as follows:
\(\frac{d_p}{t_w} ≤ \frac{82}{\sqrt{\frac{f_y}{250}}}\rightarrow V_u = V_w = 0.6*f_y*A_w\)
For a Circular Hollow Section Vv = Vw = 0.36*fy*A (not impacted by section slenderness).
When the web of the section is slender capacity is calculated as follows:
\(\frac{d_p}{t_w} > \frac{82}{\sqrt{\frac{f_y}{250}}}\rightarrow V_u = V_b = α_v*V_w\)
\(α_v = \left[\frac{82}{(\frac{d_p}{t_w})\sqrt{\frac{f_y}{250}}}\right]^2\)
Where dp is the clear depth of the web panel (i.e. depth excluding flanges), tw is the thickness of the web panel, fy is the web yield strength and Aw is the gross sectional area of the web. Note Aw is calculated differently for welded and hot rolled sections. For hot rolled sections, Aw takes the web depth as the entire section depth (d). For welded sections, Aw only takes the clear web depth between the flanges (dp). Rectangular Hollow Sections also use dp for calculation of Aw.
Non-Uniform Shear Stress Distribution
The shear capacity of a section with uniform shear stress distribution (Vv) is calculated as follows:
\(V_v = \frac{2*V_u}{0.9+\left(\frac{f*_{vm}}{f*_{va}}\right)} ≤ V_u\)
Where Vu is the section shear capacity with a uniform shear stress distribution and f*vm /f*va is the ratio of maximum and average design shear stresses in the web.
Calculating Shear Capacity in SkyCiv AS 4100 Steel Member Design
The SkyCiv AS 4100:2020 Steel Member Design tool calculates the shear capacity of a section in both principal axes. Minor axis (Z) shear capacity is calculated using the contribution of the section flanges, excluding any contribution from the section web. Results from the shear capacity calculations for a 200 UB 22.3 are detailed below.

Compression
Section Compression Capacity
AS 4100 calculates the compression capacity (Ns) of a concentrically loaded section as follows:
\(N_s = k_f*A_n*f_y\)
Where kf is the form factor of the section, An is the net area of the cross section (gross area excluding penetrations/holes) and fy is the yield strength of the section. The form factor of a section represents how much of a section can contribute to its compression capacity before local buckling occurs. The form factor is calculated as follows:
\(k_f = \frac{A_e}{A_g}\)
Where Ag is the gross area of the section, and Ae is the “effective area” of the section, i.e. the gross area of the section minus any “ineffective” areas under compression. An ineffective area is part of the section that will buckle before it reaches its yield capacity under compression. Effective areas are calculated by finding the “effective width” of each flat plate element within a section and recalculating the section area using these adjusted width values. The effective width of a flat plate element is calculated as follows:
\(b_e = b\left(\frac{λ_{ey}}{λ_{e}}\right) ≤ b\)
Where:
\(λ_e = \frac{b}{t}\sqrt{\frac{f_y}{250}}\)
Note, most design software (including SkyCiv AS 4100:2020 Steel Member Design) uses the section yield strength for element slenderness calculations, rather than the specific yield strength of the web/flange. This will always provide a conservative result. The b values used for λe calculation are identical to the dimensions used for bending section slenderness checks (with the flange split about the web), but the b used for be calculation is the total flange/web width. λey is taken from AS 4100 Table 6.2.4, depending on the edge support and residual stresses of that element.
The effective width of a circular hollow section is calculated as follows:
\(d_e = min(d_{o}\sqrt{\left(\frac{λ_{ey}}{λ_{e}}\right)}, d_{o}\left(\frac{3*λ_{ey}}{λ_{e}}\right)^2) ≤ d_{o}\)
Where:
\(λ_e = \left(\frac{d_o}{t}\right)\left(\frac{f_y}{250}\right)\)
Calculating Section Compression Capacity in SkyCiv AS 4100 Steel Member Design
The SkyCiv AS 4100:2020 Steel Member Design tool calculates the form factor and section compression capacity (Ns) for standard Australian sections and custom user-defined sections. Results from the section compression capacity calculations for a 610UB 125 are detailed below.

Tension
Section Tension Capacity
AS 4100 calculates the capacity of a tension member (Nt) as follows:
\(N_t = min(A_{g}*f_{y}\; ,\; 0.85*k_t*A_n*f_u)\)
Where Ag is the gross area of the section, An is the net area of the cross section (gross area excluding penetrations/holes), fy is the yield strength of the section, fu is the tensile (ultimate) strength of the section and kt is the tensile force distribution correction factor. The kt used in design varies depending on the section shape and connection type. Connections which provide uniform force distribution result in a kt factor of 1.0, connections with unequal force distribution result in a kt factor between 0.75-1.0.
Calculating Tension Capacity in SkyCiv AS 4100 Steel Member Design
The SkyCiv AS 4100:2020 Steel Member Design tool allows users to specify the section kt value for used in design. A lower kt value will result in a lower section tension capacity. The SkyCiv AS 4100 Member Design calculator assumes no significant holes are present in the section, hence An is taken as equal to Ag. Results from the section tension capacity calculations for a 610UB 125 are detailed below.

Member Capacity
Bending
Member Bending Moment Capacity
The bending moment capacity of a steel member may not always be governed by the bending moment capacity of the section (Ms). This is because members can fail by another method before the capacity of the section is reached. Lateral torsional buckling is a common failure method for long/unrestrained steel members, which occurs when the section rotates away from its major axis (towards its minor axis) reducing its moment capacity in the direction of bending.

AS 4100 contains guidance on calculating nominal member capacity (Mb), which factors the section capacity of a steel member (Ms) to account for the impact of member slenderness and restraint conditions.
Members with Full Lateral Restraint
Critical Flange
The critical flange of a cross-section is the flange which would deflect the furthest during buckling, eventually resulting in lateral torsional buckling failure. This is typically the compression flange of a member. Critical flange locations for standard sections under vertical loading are shown below.

Full Lateral Restraint
Shorter members with high rotational/lateral rigidity are less likely to rotate out of plane under loading, reducing the likelihood of lateral torsional buckling failure. If a member is short/rigid enough it will be able to reach its section moment capacity (Ms) before another failure method occurs. Members which satisfy this condition are deemed as having “Full Lateral Restraint”.
\(Full \; Lateral \; Restraint \; \rightarrow M_b = M_s\)
AS 4100 Clause 5.3.2 provides guidance on calculating the Full Lateral Restraint limit for a member. Circular Hollow Sections (CHS) and Square Hollow Sections (SHS) are not susceptible to lateral torsional buckling, as they have high lateral/torsional rigidity and equal section moment capacities about both axes. Hence these sections are generally assumed to achieve Full Lateral Restraint regardless of member length.
Continuous Lateral Restraint
Members which have continuous restraint to the critical flange along their entire length are deemed as having “Continuous Lateral Restraint”. Continuous Lateral Restraint is considered equivalent to Full Lateral Restraint for the calculation of member bending capacity (Mb).
Members without Full Lateral Restraint
The bending moment capacity of a member that doesn’t achieve full lateral restraint is calculated as follows:
\(M_b = α_m*α_s*M_s ≤ M_s\)
Where αm is the moment modification factor and αs is the slenderness reduction factor. AS 4100 Clause 5.6 outlines the procedure for calculating αm and αs.
Minor Axis Member Bending Capacity
The bending capacity for a member bent about its minor axis (Mb) is equal to the minor axis section capacity (Ms) about that axis. The minor axis section capacity reflects is the minimum capacity that section can achieve about any axis, hence the member cannot rotate from this axis into a less-favourable orientation.
Calculating Member Bending Capacity in SkyCiv AS 4100 Steel Member Design
The SkyCiv AS 4100:2020 Steel Member Design tool calculates carries out full lateral restraint checks and calculates member bending moment capacities about both principal axes for positive and negative bending. Users also have the option of selecting “Continuous Lateral Restraint” to bypass the Full Lateral Restraint check. Results from the member bending capacity calculations for a 3m long 200UB22.3 are detailed below.

Note, this calculator assumes αm = 1.0 and βs = -1.0 in all calculations. Cantilevered members are not supported by this tool.
Compression
Member Compression Capacity
The axial compression capacity of a member is also affected by its length, lateral rigidity and restraint conditions. Unrestrained, longer members are likely to fail due to flexural buckling before the section (squash) capacity is reached. AS 4100 contains guidance on calculating nominal member capacity (Nc), which factors the compression section capacity (Ns) to account for the impact of member slenderness and restraint conditions.
\(N_c = α_c*N_s ≤ N_s\)
Where αc is the member slenderness reduction factor. Clause 6.3.3 of AS 4100 provides guidance on calculating αc. Member compression capacity must be checked about both axes to find the governing value.
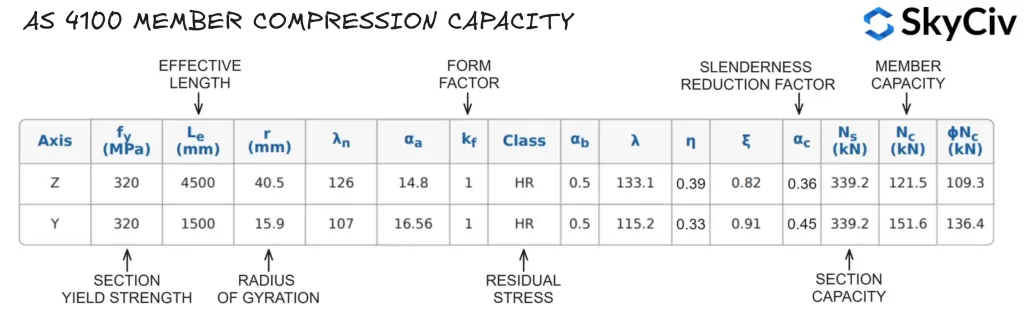
Calculating Member Compression Capacity in SkyCiv AS 4100 Steel Member Design
The SkyCiv AS 4100:2020 Steel Member Design tool calculates member compression capacity about both principal axes based on restraint lengths and effective length factors specified by the user. Results from the member compression capacity calculations for a 200UB22.3 with an unrestrained length of 4500mm and 1500mm in the Z and Y axis (respectively) are detailed below.
SkyCiv Structural Design Software
SkyCiv offers a wide range of Structural Analysis and Engineering Design Software, including:
- AS/NZS 1664 Aluminium Design
- AS/NZS 4600 Purlin Design
- AS 3600 Concrete Shear Wall Design
- AS 2870 Residential Slab on Grade Design
- AS/NZS 1576 Scaffolding Design
- AS 4055 Wind Load Calculator
Software Developer | Structural Engineer
BEng (Civil), DipEng (Software)



