Base Plate Design Example using CSA S16:19 and CSA A23.3:19
Προβληματική δήλωση:
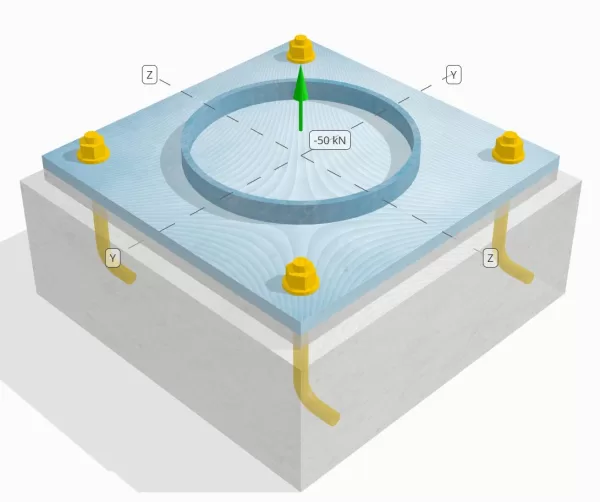
Determine whether the designed column-to-base plate connection is sufficient for a 50-kN tension load.
Δεδομένα:
Στήλη:
Ενότητα στήλης: HS324X9.5
Επιφάνεια στήλης: 9410 χιλ2
Υλικό στήλης: 230σολ
Πλάκα βάσης:
Διαστάσεις πλάκας βάσης: 500 mm x 500 χιλ
Πάχος πλάκας βάσης: 20 χιλ
Υλικό πλάκας βάσης: 230σολ
Πηκτώ:
Πάχος ενέματα: 20 χιλ
Σκυρόδεμα:
Διαστάσεις σκυροδέματος: 550 mm x 550 χιλ
Πάχος σκυροδέματος: 200 χιλ
Σκυρόδεμα: 20.68 MPa
Ραγισμένα ή αδιευκρίνιστα: Ραγισμένος
Άγκυρες:
Διάμετρος άγκυρας: 19.1 χιλ
Αποτελεσματικό μήκος ενσωμάτωσης: 130.0 χιλ
Hook length: 60χιλ
Anchor offset distance from face of column: 120.84 χιλ
Συγκολλήσεις:
Weld type: CJP
Η ταξινόμηση μετάλλων πλήρωσης: E43xx
Δεδομένα αγκυροβόλησης (από Υπολογιστής Skyciv):

Ορισμοί:
Διαδρομή φόρτωσης:
Όταν μια πλάκα βάσης υποβάλλεται σε ανύψωση (εντάσεως) δυνάμεις, Αυτές οι δυνάμεις μεταφέρονται στις ράβδους άγκυρας, που με τη σειρά τους προκαλούν στιγμές κάμψης στην πλάκα βάσης. Η δράση κάμψης μπορεί να απεικονιστεί ως κάμψη που συμβαίνουν γύρω από τις φλάντζες ή τον ιστό της ενότητας στήλης, ανάλογα με το πού τοποθετούνται οι άγκυρες.
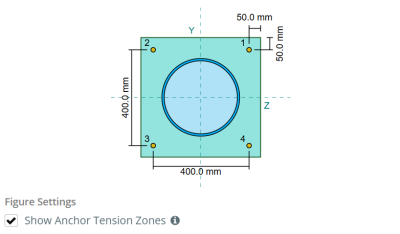
Στο Λογισμικό σχεδιασμού πλάκας βάσης SkyCIV, μόνο άγκυρες που βρίσκονται μέσα στο ζώνη τάσης άγκυρας θεωρούνται αποτελεσματικοί στην αντιστάθμιση της ανύψωσης. Αυτή η ζώνη περιλαμβάνει συνήθως περιοχές κοντά στις φλάντζες της στήλης ή στο διαδίκτυο. In the case of a circular column, the anchor tension zone includes the entire area outside the column perimeter. Οι άγκυρες εκτός αυτής της ζώνης δεν συμβάλλουν στην αντίσταση στην ένταση και αποκλείονται από τους υπολογισμούς ανύψωσης.
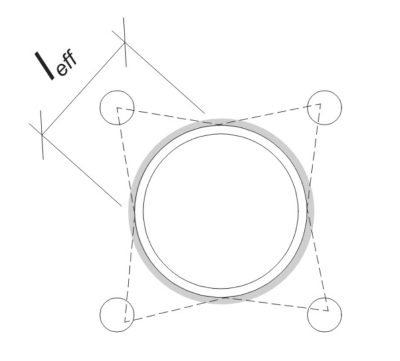
Για να προσδιορίσετε την αποτελεσματική περιοχή της πλάκας βάσης που αντιστέκεται στην κάμψη, ένα 45-διασπορά υποτίθεται από την κεντρική γραμμή κάθε ράβδου αγκύρωσης προς την επιφάνεια της στήλης. Αυτή η διασπορά ορίζει το αποτελεσματικό μήκος συγκόλλησης και βοηθά στη δημιουργία του αποτελεσματικό πλάτος κάμψης του πιάτου.
Η υπόθεση απλοποιεί την ανάλυση της πλάκας βάσης προσεγγίζοντας τον τρόπο με τον οποίο εξαπλώνεται η δύναμη ανύψωσης μέσω της πλάκας.
Ομάδες άγκυρας:
ο Λογισμικό σχεδιασμού πλάκας βάσης SkyCIV Περιλαμβάνει ένα διαισθητικό χαρακτηριστικό που προσδιορίζει ποιες άγκυρες αποτελούν μέρος μιας ομάδας αγκύρωσης για αξιολόγηση ξέσπασμα σκυροδέματος και concrete side-face blowout αποτυχία.
Ενα ομάδα άγκυρας Αποτελείται από πολλαπλές άγκυρες με παρόμοια αποτελεσματικά βάθη ενσωμάτωσης και απόσταση, και είναι αρκετά κοντά ώστε τους Οι προβλεπόμενες περιοχές αντίστασης επικαλύπτονται. Όταν ομαδοποιούνται άγκυρες, Οι ικανότητές τους συνδυάζονται για να αντισταθούν στη συνολική δύναμη έντασης που εφαρμόζεται στην ομάδα.
Οι άγκυρες που δεν πληρούν τα κριτήρια ομαδοποίησης αντιμετωπίζονται ως άγκυρες. Σε αυτήν την περίπτωση, Μόνο η δύναμη τάσης στην ατομική άγκυρα ελέγχεται από τη δική της αποτελεσματική περιοχή αντίστασης.
Υπολογισμοί βήμα προς βήμα:
Ελεγχος #1: Υπολογίστε τη χωρητικότητα συγκόλλησης
Να ξεκινήσω, we need to calculate the load per anchor and determine the effective weld length for each anchor. ο αποτελεσματικό μήκος συγκόλλησης is based on a 45° dispersion line drawn from the center of the anchor to the face of the column. If this 45° line does not intersect the column, ο tangent points are used instead. Επιπροσθέτως, if the anchors are closely spaced, the effective weld length is reduced to avoid overlap. Τελικά, the sum of all effective weld lengths must not exceed the actual weldable length available along the column circumference.
Let’s apply this to our example. Based on the given geometry, the 45° line from the anchor does not intersect the column. Σαν άποτέλεσμα, the arc length between the tangent points is used instead. This arc length must also account for any adjacent anchors, with any overlapping portions subtracted to avoid double-counting. The calculated arc length is:
\(
μεγάλο_{\κείμενο{τόξο}} = 254.47 \, \κείμενο{χιλ}
\)
This arc length calculation is fully automated in the SkyCiv Base Plate Design Software, but it can also be performed manually using trigonometric methods. You can try the free tool from this link.
Considering the available weldable length along the column’s circumference, the final αποτελεσματικό μήκος συγκόλλησης είναι:
\(
μεγάλο_{\κείμενο{εφ}} = min αριστερά( μεγάλο_{\κείμενο{τόξο}}, \frac{\pi d_{\κείμενο{διάσελο}}}{n_{ένα,τ}} \σωστά) = min αριστερά( 254.47 \, \κείμενο{χιλ}, \frac{\pi \times 324 \, \κείμενο{χιλ}}{4} \σωστά) = 254.47 \, \κείμενο{χιλ}
\)
Επόμενο, ας υπολογίσουμε το φορτίο ανά άγκυρα. Για ένα δεδομένο σύνολο τεσσάρων (4) άγκυρες, Το φορτίο ανά άγκυρα είναι:
\(
T_{εσύ,\κείμενο{άγκυρα}} = frac{N_x}{n_{ένα,τ}} = frac{50 \, \κείμενο{ΚΝ}}{4} = 12.5 \, \κείμενο{ΚΝ}
\)
Χρήση του υπολογισμένου αποτελεσματικού μήκους συγκόλλησης, we can now compute the required force per unit length acting on the weld.
\(
v_f = \frac{T_{εσύ,\κείμενο{άγκυρα}}}{μεγάλο_{\κείμενο{εφ}}} = frac{12.5 \, \κείμενο{ΚΝ}}{254.47 \, \κείμενο{χιλ}} = 0.049122 \, \κείμενο{kN / mm}
\)
Τώρα, we refer to CSA S16:19 Ρήτρα 13.13.3.1 to calculate the factored resistance of the complete joint penetration (CJP) συγκόλληση. This requires the base metal resistance, expressed in force per unit length, for both the column and the base plate materials.
\(
v_{ρ,\κείμενο{bm}} = phi αριστερά( \min left( ΦΑ_{και,\κείμενο{διάσελο}} αυτό είναι ένα πολύ σημαντικό στάδιο στο σχεδιασμό ενός τοίχου αντιστήριξης, καθώς η μη αντιστοίχιση των σωστών αναλογικών διαστάσεων από την αρχή σε κάθε στοιχείο μπορεί να οδηγήσει στην ανάγκη πολλών επαναλήψεων για να συμμορφωθεί ο τοίχος αντιστήριξης με τις απαιτήσεις ευστάθειας ή υπερμεγέθη σύστημα που πληροί όλες τις απαιτήσεις, αλλά χρησιμοποιεί πολύ περισσότερο υλικό από το θεωρητικό ελάχιστο{\κείμενο{διάσελο}}, ΦΑ_{και,\κείμενο{bp}} αυτό είναι ένα πολύ σημαντικό στάδιο στο σχεδιασμό ενός τοίχου αντιστήριξης, καθώς η μη αντιστοίχιση των σωστών αναλογικών διαστάσεων από την αρχή σε κάθε στοιχείο μπορεί να οδηγήσει στην ανάγκη πολλών επαναλήψεων για να συμμορφωθεί ο τοίχος αντιστήριξης με τις απαιτήσεις ευστάθειας ή υπερμεγέθη σύστημα που πληροί όλες τις απαιτήσεις, αλλά χρησιμοποιεί πολύ περισσότερο υλικό από το θεωρητικό ελάχιστο{\κείμενο{bp}} \σωστά) \σωστά)
\)
\(
v_{ρ,\κείμενο{bm}} = 0.9 \φορές αριστερά( \min left( 230 \, \κείμενο{MPa} \φορές 9.53 \, \κείμενο{χιλ}, 230 \, \κείμενο{MPa} \φορές 20 \, \κείμενο{χιλ} \σωστά) \σωστά) = 1.9727 \, \κείμενο{kN / mm}
\)
Από 0.049122 kN / mm < 1.9727 kN / mm, Η χωρητικότητα συγκόλλησης είναι επαρκής.
Ελεγχος #2: Υπολογίστε τη χωρητικότητα κάμψης πλάκας βάσης λόγω φορτίου τάσης
Using the load per anchor and the offset distance from the center of the anchor to the face of the column, Η στιγμή που εφαρμόζεται στην πλάκα βάσης μπορεί να υπολογιστεί χρησιμοποιώντας ένα υποστήριγμα υπόθεση. For a circular column, the load eccentricity is determined by considering the sagitta of the welded arc, and can be calculated as follows:
\(
μι_{\κείμενο{pipe}} = d_o + r_{\κείμενο{διάσελο}} \αριστερά( 1 – \cos αριστερά( \frac{μεγάλο_{\κείμενο{εφ}}}{2 r_{\κείμενο{διάσελο}}} \σωστά) \σωστά)
\)
\(
μι_{\κείμενο{pipe}} = 120.84 \, \κείμενο{χιλ} + 162 \, \κείμενο{χιλ} \φορές αριστερά( 1 – \cos αριστερά( \frac{254.47 \, \κείμενο{χιλ}}{2 \φορές 162 \, \κείμενο{χιλ}} \σωστά) \σωστά) = 168.29 \, \κείμενο{χιλ}
\)
The induced moment is computed as:
\(
M_f = T_{εσύ,\κείμενο{άγκυρα}} μι_{\κείμενο{pipe}} = 12.5 \, \κείμενο{ΚΝ} \φορές 168.29 \, \κείμενο{χιλ} = 2103.6 \, \κείμενο{ΚΝ} \cdot \text{χιλ}
\)
Επόμενο, we will determine the bending width of the base plate. Για αυτό, we use the chord length corresponding to the effective weld arc.
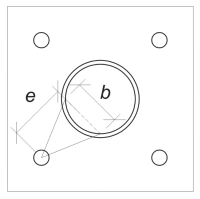
\(
\theta_{\κείμενο{rad}} = frac{μεγάλο_{\κείμενο{εφ}}}{0.5 ρε_{\κείμενο{διάσελο}}} = frac{254.47 \, \κείμενο{χιλ}}{0.5 \φορές 324 \, \κείμενο{χιλ}} = 1.5708
\)
\(
b = d_{\κείμενο{διάσελο}} \αριστερά( \αμαρτία αριστερά( \frac{\theta_{\κείμενο{rad}}}{2} \σωστά) \σωστά) = 324 \, \κείμενο{χιλ} \φορές αριστερά( \αμαρτία αριστερά( \frac{1.5708}{2} \σωστά) \σωστά) = 229.1 \, \κείμενο{χιλ}
\)
Τελικά, Μπορούμε να υπολογίσουμε το factored flexural resistance της πλάκας βάσης χρησιμοποιώντας CSA S16:19 Ρήτρα 13.5.
\(
M_r = \phi F_{και,\κείμενο{bp}} Z_{\κείμενο{εφ}} = 0.9 \φορές 230 \, \κείμενο{MPa} \φορές 22910 \, \κείμενο{χιλ}^3 = 4742.4 \, \κείμενο{ΚΝ} \cdot \text{χιλ}
\)
Οπου,
\(
Z_{\κείμενο{εφ}} = frac{σι (αυτό είναι ένα πολύ σημαντικό στάδιο στο σχεδιασμό ενός τοίχου αντιστήριξης, καθώς η μη αντιστοίχιση των σωστών αναλογικών διαστάσεων από την αρχή σε κάθε στοιχείο μπορεί να οδηγήσει στην ανάγκη πολλών επαναλήψεων για να συμμορφωθεί ο τοίχος αντιστήριξης με τις απαιτήσεις ευστάθειας ή υπερμεγέθη σύστημα που πληροί όλες τις απαιτήσεις, αλλά χρησιμοποιεί πολύ περισσότερο υλικό από το θεωρητικό ελάχιστο{\κείμενο{bp}})^ 2}{4} = frac{229.1 \, \κείμενο{χιλ} \φορές (20 \, \κείμενο{χιλ})^ 2}{4} = 22910 \, \κείμενο{χιλ}^ 3
\)
Από 2103.6 kN-mm < 4742.4 kN-mm, Η ικανότητα απόδοσης της κάμψης της πλάκας βάσης είναι επαρκής.
Ελεγχος #3: Υπολογίστε την ικανότητα εφελκυσμού της ράβδου άγκυρας
Για να αξιολογηθεί η ικανότητα εφελκυσμού της ράβδου αγκύρωσης, we refer to CSA A23.3:19 Clause D.6.1.2 and CSA S16:19 Ρήτρα 25.3.2.1.
Πρώτα, καθορίζουμε το συγκεκριμένη αντοχή εφελκυσμού του χάλυβα άγκυρας. Αυτή είναι η χαμηλότερη τιμή που επιτρέπεται από CSA A23.3:19 Clause D.6.1.2.
\(
φά_{\κείμενο{Γιούτα}} = min αριστερά( ΦΑ_{εσύ,\κείμενο{Α.Κ.}}, 1.9 ΦΑ_{και,\κείμενο{Α.Κ.}}, 860 \σωστά) = min αριστερά( 400 \, \κείμενο{MPa}, 1.9 \φορές 248.2 \, \κείμενο{MPa}, 860.00 \, \κείμενο{MPa} \σωστά) = 400 \, \κείμενο{MPa}
\)
Επόμενο, καθορίζουμε το αποτελεσματική περιοχή διατομής of the anchor rod in tension using CAC Concrete Design Handbook, 3έκδοση, Τραπέζι 12.3.
\(
ΕΝΑ_{Ξέρω,Ν} = 215 \, \κείμενο{χιλ}^ 2
\)
Με αυτές τις τιμές, Εφαρμόζουμε CSA A23.3:19 Εξ. D.2 Για να υπολογίσετε το factored tensile resistance της ράβδου άγκυρας.
\(
Ν_{\κείμενο{sar}} = Α_{Ξέρω,Ν} \phi_s f_{\κείμενο{Γιούτα}} R = 215 \, \κείμενο{χιλ}^2 φορές 0.85 \φορές 400 \, \κείμενο{MPa} \φορές 0.8 = 58.465 \, \κείμενο{ΚΝ}
\)
Επιπροσθέτως, Αξιολογούμε το factored tensile resistance according to CSA S16:19 Ρήτρα 25.3.2.1.
\(
T_r = \phi_{Με} 0.85 ΕΝΑ_{Με} ΦΑ_{εσύ,\κείμενο{Α.Κ.}} = 0.67 \φορές 0.85 \φορές 285.02 \, \κείμενο{χιλ}^2 φορές 400 \, \κείμενο{MPa} = 64.912 \, \κείμενο{ΚΝ}
\)
After comparing the two, we identify that the factored resistance calculated using CSA A23.3:19 governs in this case.
Θυμηθείτε τους προηγουμένως υπολογισμένους φορτίο έντασης ανά άγκυρα:
\(
Ν_{fa} = frac{N_x}{n_{ένα,τ}} = frac{50 \, \κείμενο{ΚΝ}}{4} = 12.5 \, \κείμενο{ΚΝ}
\)
Από 12.5 ΚΝ < 58.465 ΚΝ, Η χωρητικότητα εφελκυσμού της ράβδου άγκυρας είναι επαρκής.
Ελεγχος #4: Υπολογίστε τη χωρητικότητα ξεμπλοκάρισμα από σκυρόδεμα σε ένταση

Πριν υπολογίσετε την χωρητικότητα ξεμπλοκάρισμα, πρέπει πρώτα να καθορίσουμε εάν το μέλος χαρακτηρίζεται ως στενό μέλος. Σύμφωνα με CSA A23.3:19 Clause D.6.2.3, the member does not meet the criteria for a narrow member. Επομένως, the given effective embedment length will be used in the calculations.
Χρησιμοποιώντας CSA A23.3:19 Εξ. D.5, υπολογίζουμε το Μέγιστη επιφάνεια κώνου σκυροδέματος για μια μόνο άγκυρα, based on the effective embedment length.
\(
ΕΝΑ_{Θυμάμαι} = 9 (ω_{εφ,s1})💕⬛ Αγορά Indocin από 9 \φορές (130 \, \κείμενο{χιλ})💕⬛ Αγορά Indocin από 152100 \, \κείμενο{χιλ}^ 2
\)
Ομοίως, we use the effective embedment length to calculate the Πραγματική προβλεπόμενη περιοχή κώνου σκυροδέματος of the single anchor.
\(
ΕΝΑ_{Αρ} = L_{Αρ} ΣΙ_{Αρ} = 270 \, \κείμενο{χιλ} \φορές 270 \, \κείμενο{χιλ} = 72900 \, \κείμενο{χιλ}^ 2
\)
Οπου,
\(
ΜΕΓΑΛΟ_{Αρ} = αριστερά( \min left( ντο_{\κείμενο{αριστερά},s1}, 1.5 ω_{εφ,s1} \σωστά) \σωστά) + \αριστερά( \min left( ντο_{\κείμενο{σωστά},s1}, 1.5 ω_{εφ,s1} \σωστά) \σωστά)
\)
\(
ΜΕΓΑΛΟ_{Αρ} = αριστερά( \min left( 475 \, \κείμενο{χιλ}, 1.5 \φορές 130 \, \κείμενο{χιλ} \σωστά) \σωστά) + \αριστερά( \min left( 75 \, \κείμενο{χιλ}, 1.5 \φορές 130 \, \κείμενο{χιλ} \σωστά) \σωστά)
\)
\(
ΜΕΓΑΛΟ_{Αρ} = 270 \, \κείμενο{χιλ}
\)
\(
ΣΙ_{Αρ} = αριστερά( \min left( ντο_{\κείμενο{μπλουζα},s1}, 1.5 ω_{εφ,s1} \σωστά) \σωστά) + \αριστερά( \min left( ντο_{\κείμενο{κάτω μέρος},s1}, 1.5 ω_{εφ,s1} \σωστά) \σωστά)
\)
\(
ΣΙ_{Αρ} = αριστερά( \min left( 75 \, \κείμενο{χιλ}, 1.5 \φορές 130 \, \κείμενο{χιλ} \σωστά) \σωστά) + \αριστερά( \min left( 475 \, \κείμενο{χιλ}, 1.5 \φορές 130 \, \κείμενο{χιλ} \σωστά) \σωστά)
\)
\(
ΣΙ_{Αρ} = 270 \, \κείμενο{χιλ}
\)
Επόμενο, Αξιολογούμε το factored basic concrete breakout resistance μιας μόνο άγκυρας που χρησιμοποιεί CSA A23.3:19 Εξ. D.6
\(
Ν_{br} = k_c \phi \lambda_a \sqrt{\frac{f'_c}{\κείμενο{MPa}}} \αριστερά( \frac{ω_{εφ,s1}}{\κείμενο{χιλ}} \σωστά)^{1.5} R N
\)
\(
Ν_{br} = 10 \φορές 0.65 \φορές 1 \φορές sqrt{\frac{20.68 \, \κείμενο{MPa}}{1 \, \κείμενο{MPa}}} \φορές αριστερά( \frac{130 \, \κείμενο{χιλ}}{1 \, \κείμενο{χιλ}} \σωστά)^{1.5} \φορές 1 \φορές 0.001 \, \κείμενο{ΚΝ} = 43.813 \, \κείμενο{ΚΝ}
\)
Οπου,
- \(κ_{ντο} = 10\) για άγκυρες
- \(\lambda = 1.0 \) Για σκυρόδεμα κανονικού βάρους
Τώρα, Αξιολογούμε τις επιδράσεις της γεωμετρίας με τον υπολογισμό του συντελεστής ακμής.
Η συντομότερη απόσταση ακμής της ομάδας αγκύρωσης καθορίζεται ως:
\(
ντο_{ένα,\κείμενο{ελάχ}} = min αριστερά( ντο_{\κείμενο{αριστερά},s1}, ντο_{\κείμενο{σωστά},s1}, ντο_{\κείμενο{μπλουζα},s1}, ντο_{\κείμενο{κάτω μέρος},s1} \σωστά) = min αριστερά( 475 \, \κείμενο{χιλ}, 75 \, \κείμενο{χιλ}, 75 \, \κείμενο{χιλ}, 475 \, \κείμενο{χιλ} \σωστά) = 75 \, \κείμενο{χιλ}
\)
Σύμφωνα με CSA A23.3:19 Εξ. D.10 and D.11, το ξεμπλοκάρισμα συντελεστής ακμής είναι:
\(
\Psi_{εκδ,Ν} = min αριστερά( 1.0, 0.7 + 0.3 \αριστερά( \frac{ντο_{ένα,\κείμενο{ελάχ}}}{1.5 ω_{εφ,s1}} \σωστά) \σωστά) = min αριστερά( 1, 0.7 + 0.3 \φορές αριστερά( \frac{75 \, \κείμενο{χιλ}}{1.5 \φορές 130 \, \κείμενο{χιλ}} \σωστά) \σωστά) = 0.81538
\)
Επιπλέον, και οι δύο συντελεστής πυρόλυσης και το συντελεστής διαίρεσης λαμβάνονται ως:
\(
\Psi_{ντο,Ν} = 1
\)
\(
\Psi_{cp,Ν} = 1
\)
Τότε, Συνδυάζουμε όλους αυτούς τους παράγοντες και τη χρήση ACI 318-19 Εξ. 17.6.2.1σι Για να αξιολογήσετε το factored concrete breakout resistance of the single anchor:
\(
Ν_{cbr} = αριστερά( \frac{ΕΝΑ_{Αρ}}{ΕΝΑ_{Θυμάμαι}} \σωστά) \Psi_{εκδ,Ν} \Psi_{ντο,Ν} \Psi_{cp,Ν} Ν_{br} = αριστερά( \frac{72900 \, \κείμενο{χιλ}^ 2}{152100 \, \κείμενο{χιλ}^ 2} \σωστά) \φορές 0.81538 \φορές 1 \φορές 1 \φορές 43.813 \, \κείμενο{ΚΝ} = 17.122 \, \κείμενο{ΚΝ}
\)
Θυμηθείτε τους προηγουμένως υπολογισμένους φορτίο έντασης ανά άγκυρα:
\(
Ν_{fa} = frac{N_x}{n_{ένα,μικρό}} = frac{50 \, \κείμενο{ΚΝ}}{4} = 12.5 \, \κείμενο{ΚΝ}
\)
Από 12.5 ΚΝ < 17.122 ΚΝ Η χωρητικότητα ξεμπλοκάρισμα του σκυροδέματος είναι επαρκής.
This concrete breakout calculation is based on Anchor ID #1. The same capacity will apply to the other anchors due to the symmetric design.
Ελεγχος #5: Υπολογίστε την χωρητικότητα αγκύρωσης
Η ικανότητα απομάκρυνσης μιας άγκυρας διέπεται από την αντίσταση στο ενσωματωμένο άκρο της. For hooked anchors, it is dependent on its hook length.
We compute the factored basic anchor pullout resistance ανά CSA A23.3:19 Εξ. D.17.
\(
Ν_{πρ} = \Psi_{ντο,Π} 0.9 \phi (f'_c) e_h d_a R = 1 \φορές 0.9 \φορές 0.65 \φορές (20.68 \, \κείμενο{MPa}) \φορές 60 \, \κείμενο{χιλ} \φορές 19.05 \, \κείμενο{χιλ} \φορές 1 = 13.828 \, \κείμενο{ΚΝ}
\)
Θυμηθείτε τους προηγουμένως υπολογισμένους φορτίο έντασης ανά άγκυρα:
\(
Ν_{fa} = frac{N_x}{n_{ένα,τ}} = frac{50 \, \κείμενο{ΚΝ}}{4} = 12.5 \, \κείμενο{ΚΝ}
\)
Από 12.5 ΚΝ < 13.828 ΚΝ, η χωρητικότητα αγκύρωσης είναι επαρκής.
Ελεγχος #6: Υπολογίστε την χωρητικότητα εκτόξευσης πλευρικού προσώπου σε κατεύθυνση y
This calculation is not applicable for hooked anchors.
Ελεγχος #7: Υπολογίστε την χωρητικότητα εκτόξευσης πλευρικού προσώπου στην κατεύθυνση z
This calculation is not applicable for hooked anchors.
Περίληψη σχεδίου
ο Λογισμικό σχεδιασμού πλάκας βάσης SkyCIV Μπορεί να δημιουργήσει αυτόματα μια αναφορά υπολογισμού βήμα προς βήμα για αυτό το παράδειγμα σχεδιασμού. Παρέχει επίσης μια περίληψη των επιταγών που εκτελούνται και των προκύπτουσων αναλογιών τους, καθιστώντας τις πληροφορίες κατανοητές με μια ματιά. Παρακάτω είναι ένας πίνακας συνοπτικών δείγματος, που περιλαμβάνεται στην αναφορά.
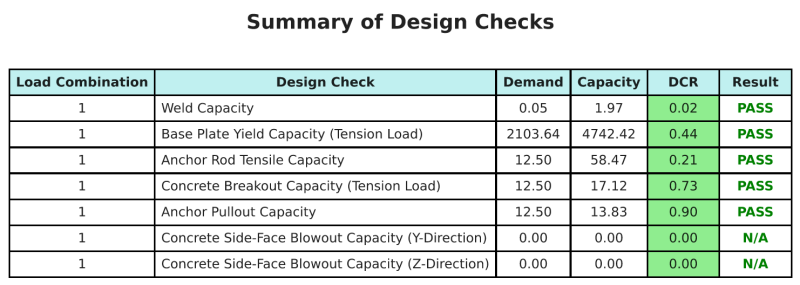
Αναφορά δείγματος SkyCIV
Sample report will be added soon.
Αγορά λογισμικού πλάκας βάσης
Purchase the full version of the base plate design module onits own without any other SkyCiv modules. Αυτό σας δίνει ένα πλήρες σύνολο αποτελεσμάτων για σχεδιασμό πλάκας βάσης, συμπεριλαμβανομένων λεπτομερών αναφορών και περισσότερων λειτουργιών.