Estimating Pile Capacity
Estimating the Pile load-carrying capacity is necessary to determine the ultimate axial load that the pile can carry. The ultimate load capacity of the pile (Ερ) is equivalent to the sum of end-bearing capacity (Qp) and frictional resistance (Qs), represented by Fig. 1 and Eq. 1. Numerous published studies and practices determine the pile’s end-bearing capacity and frictional resistance. This article focuses on various methods to estimate the ultimate pile capacity.
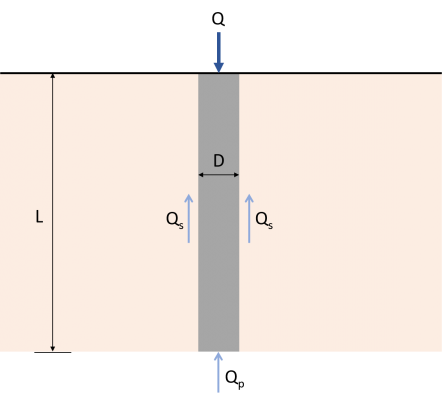
\( {Ερ}_{εσύ} = {Ερ}_{Π} + {Ερ}_{μικρό} \) (1)
Ερεσύ : Ultimate load-carrying capacity
ΕρΠ : End-bearing load capacity
Ερμικρό : Skin-frictional resistance
Universal equations for QΠ και Qμικρό
\( {Ερ}_{Π} = {ΕΝΑ}_{Π} \φορές {ε}_{Π} \) (2)
\( {ε}_{Π} = (c \times {Ν}_{ντο}) + (ε’ \φορές {Ν}_{ε}) + (\gamma \times D \times {Ν}_{\gamma}) \) (3)
\( {Ερ}_{Π} = {ΕΝΑ}_{Π} \φορές[ (c \times {Ν}_{ντο}) + (ε’ \φορές {Ν}_{ε}) ] \) (4)
The total frictional resistance of the pile, which is developed along its length, can be calculated using this equation:
\( {Ερ}_{μικρό} = ∑ (p × ΔL × f) \) (5)
Π: Perimeter of the pile
ΔL: Incremental pile length over which p and f are taken
φά: Unit frictional resistance at any depth
Methods for Estimating Qp
Meyerhof’s Method
Sandy Soil
According to Meyerhof, the unit point resistance (εΠ) of piles in sand generally increases with the embedment length until it reaches its maximum value when the embedment ratio (L/D) reaches a critical value. Critical embedment ratio (L/D)Ενώ το Restraint θα απαιτήσει να εισαγάγετε το usually varies from 16 προς το 18. Σε αυτή τη μέθοδο, piles in the sand are assumed to have zero cohesion (c ≈ 0), and the unit point resistance should not exceed limiting point resistance (εμεγάλο), which is given by Eq. 7. The bearing capacity factor (Nq) values are directly proportional to the soil friction angle of the bearing stratum (Τραπέζι 1). Based on Meyerhof’s theory, the universal equation for QΠ (Eq.4) can be simplified to:
\( {Ερ}_{Π} = {ΕΝΑ}_{Π} \φορές (ε’ \φορές {Ν}_{ε}) \leq ({ΕΝΑ}_{Π} \φορές {ε}_{μεγάλο}) \) (6)
\( {ε}_{μεγάλο} = 0.5 \φορές {Π}_{ένα} \φορές {Ν}_{ε} \times tan (\φι') \) (7)
εμεγάλο : Limiting point resistance
Πένα: Atmospheric pressure (≈100 kN/m2)
\( \phi’\): Effective soil friction angle at the tip of the pile
Τραπέζι 1: Interpolated values of Nε (Meyerhof’s theory)
Clay Soil
Εξίσωση 4 can also calculate the end-bearing capacity of piles in clay or cohesive soils (φ ≈ 0). Since soil friction angle is neglected and the bearing capacity factor (Νντο) has a constant value of 9 for cohesive soils, Eq.4 can be written as:
\( {Ερ}_{Π} = {ΕΝΑ}_{Π} \times c \times {Ν}_{ντο} = 9 \times c \times {ΕΝΑ}_{Π} \) (8)
Vesic’s Method
Vesic’s method of calculating end-bearing capacity on sandy or clayey soils is based on his theory of the expansion of cavities.
Sandy Soil
Based on his theory, end-bearing capacity of piles in sand can be estimated using the following equations:
\( {Ερ}_{Π} = {ΕΝΑ}_{Π} \times \bar{\sigma’}_{ο} \φορές {Ν}_{\sigma} \) (9)
\(\μπαρ{\sigma’}_{ο} = frac{1 + (2 \φορές {κ}_{ο})}{3} \times q’\) (10)
\( {κ}_{ο} = 1 – sin \phi’\) (11)
\( {Ν}_{\sigma} = frac{3 \φορές {Ν}_{ε}}{1 + (2 \φορές {κ}_{ο})} \) (12)
\(\μπαρ{\sigma’}_{ο} \) : Mean effective normal ground stress at the level of the pile point
Ko: Earth pressure coefficient at rest
Nσ: Bearing capacity factor
Clay Soil
Same with Meyerhof’s method, Εξ. 4 is also applicable to calculate the end-bearing capacity of piles in clay. Ωστόσο, the value of the bearing capacity factor (Νντο) is a factor of rigidty index (Εγώρ). According to his theory of expansion of cavities, Νντο και εγώρ can be estimated by:
\( {Ν}_{ντο} = (\frac{4}{3}) \φορές [στο({Εγώ}_{ρ}) + 1] + \frac{\πι}{2} + 1 \) (13)
\( {Εγώ}_{ρ} = frac{{μι}_{μικρό}}{3 \times c} \) (For φ ≈ 0)(14)
Εγώρ: Rigidity index
μιμικρό: Modulus of elasticity of soil
Coyle and Castello’s Method (Sandy Soil)
Based on 24 large-scale field load tests of driven piles in sand, Coyle and Castello suggested that the end-bearing capacity of piles can be calculated using Eq.15. The values of the bearing capacity factor (Nq) is a factor of both embedment ratio (L/D) and the soil friction angle (φ’), όπως φαίνεται στο Σχ. 2
\( {Ερ}_{Π} = {ΕΝΑ}_{Π} \φορές (ε’ \φορές {Ν}_{ε}) \) (15)
Φιγούρα 2: Variation of Nq with L/D & φ’ (Redrawn after Coyle & Castello, 1981)
Πηγή: ο, Braja. Αρχές Μηχανικής του Ιδρύματος (7ου Έκδοση, p.564)
Methods for Estimating Qs
Frictional Resistance of Piles in Sand
The unit frictional resistance of piles in sand, as shown in Eq. 5, considers multiple factors which are quite difficult to calculate. It includes the earth pressure coefficient (κ) & soil-pile friction angle, which both have varying values depending on which approach to use or to the available soil data.
\( f = K\times {\sigma}_{ο}’ \times tan (\delta) \) (15)
κ: Effective earth pressure coefficient
σ’ο: Effective vertical stress at the depth under consideration
δ: Soil-pile friction angle
The following are the different ways to estimate the effective earth pressure coefficient and soil-friction angle values. These variables are a factor of soil frictional angle (φ’) or pile type.
Effective earth pressure coefficient
The soil exerts lateral earth pressure to the pile surface. It is necessary to account for this pressure on the design or analysis for stability. The following are the different ways to determine the earth pressure coefficients to calculate the unit frictional resistance of piles in sand.
NAVFAC DM 7.2
| Τύπος σωρού | Συμπίεση | Uplift |
|---|---|---|
Τραπέζι 2: Earth pressure coefficient, κ (NAVFAC DM 7.2)
Average K Method
The earth pressure coefficient (κ) can also be evaluated by taking the average of earth pressure coefficient at rest (κ0), active earth pressure (κένα), and passive earth pressure (κΠ), as shown from Equations 16-19.
\( K =\frac{{κ}_{0} + {κ}_{ένα} + {κ}_{Π}}{3} \) (16)
\( (κ)_{0} =1 – sin \phi \) (17)
\( (K_{ένα} =1 – {tan}^{2}( \frac{45 – \phi}{2}) \) (18)
\( (K_{Π} =1 + {tan}^{2}( \frac{45 + \phi}{2}) \) (19)
Mansur and Hunter (1970)
Based on different field load test results, Mansur and Hunter concluded the values of earth pressure coefficient with the corresponding pile types.
| Τύπος σωρού | κ |
|---|---|
Τραπέζι 3: Earth pressure coefficient, κ (Mansur and Hunter, 1970)
Soil-pile Friction Angle
The friction angle between the soil and the surface of the pile is an essential aspect of foundation design. Practically, many engineers approximate this value as equal to 2/3 of the internal friction angle of the soil. Ωστόσο, based on the study of Coyle and Castello in 1981, the soil-pile friction angle is approximately equivalent to 80% of the internal friction angle of the soil. Αφ 'ετέρου, NAVFAC DM7.2 uses these values to estimate the friction angle between the soil and pile:
| Τύπος σωρού | δ |
|---|---|
Τραπέζι 4: Soil-pile friction angle (δ) (NAVFAC DM 7.2)
Frictional Resistance of piles in Clay
Calculating the frictional resistance of piles in clayey soils can be as challenging as the one in sandy soils due to the introduction of new variables, which are also not as easy to determine. Ωστόσο, there are several available methods to obtain the values of these variables.
λ Method
Based on the study of Vijayvergiya and Focht in 1972, the total frictional resistance of piles in clay can be estimated by determining the average unit frictional resistance of the pile, as shown by Equations 20 και 21. λ values changes as the depth of the penetration of pile increases. Τραπέζι 5 shows the variation of λ with the embedment length of the pile.
\( {φά}_{av} = \lambda \times [\μπαρ{\sigma’}_{ο} +( 2 \φορές {ντο}_{εσύ})] \) (20)
\({Ερ}_{μικρό} = p \times L \times {φά}_{av} \) (21)
\( \μπαρ{\sigma’}_{ο} \): Mean effective vertical stress for the entire embedment length
ντοεσύ: Mean undrained shear strength
| μεγάλο (Μ) | λ |
|---|---|
Τραπέζι 5: Variation of λ with pile embedment length (μεγάλο)
α Method
The α method suggests that unit frictional resistance of piles is equivalent to the product of the undrained cohesion of the soil layer and its corresponding empirical adhesion factor (α). Τραπέζι 6 shows the corresponding value of the adhesion factor with the ratio of undrained cohesion and atmospheric pressure (ντοεσύ/Πένα).
\(f = \alpha \times {ντο}_{εσύ}\) (22)
Επομένως, the total frictional resistance of pile in clay using this method can be re-written as:
\({Ερ}_{μικρό} = άθροισμα (f \times p \times \Delta L) = άθροισμα (\alpha \times {ντο}_{εσύ} \times p \times \Delta L)\) (23)
| ντοεσύ/Πένα | α |
|---|---|
| 0.8 | |
Πένα = ατμοσφαιρική πίεση ≈ 100 kN / m2
Τραπέζι 6: Variation of α (Terzaghi, Τσιμπώ, και Mesri, 1996)
β Method
Pore water pressure around the pile increases when the pile is driven into saturated clays. Αυτή η μέθοδος, based on effective stress analysis, is suited for long-term (drained) analyses of the pile load capacity as it considers the gradual dissipation of the excess pore water pressure over time. According to Tomlinson (1971), piles driven in soft clays assume that failures occur in the remolded soil close to the pile surface. Based on Eq. 15, ο όρος (K × tanδ) for unit frictional resistance of piles in sand shall be represented by β. The soil-friction angle (δ) shall be replaced by a remolded drained friction angle of the soil (Φ’Ρ). Thus the unit frictional resistance of piles in clay is estimated to be equal to:
\(f = \beta \times {\sigma’}_{ο}\) (24)
\(\beta = K \times tan {\Phi ‘}_{Ρ}\) (25)
Conservatively, the earth pressure coefficient (κ) is equivalent to the earth pressure coefficient at rest (κ0) which varies for normally consolidated clays and overconsolidated clays, as shown in the following equations:
\( Κ = {κ}_{0} = 1 – χωρίς {\Phi ‘}_{Ρ}\) (Normally consolidated clays) (26)
\( Κ = {κ}_{0} = (1 – χωρίς {\Phi ‘}_{Ρ}) \φορές sqrt(OCR)\) (Overconsolidated clays) (27)
OCR: Overconsolidation ratio
Θέλετε να δοκιμάσετε το λογισμικό Foundation Design του SkyCiv? Το δωρεάν εργαλείο μας επιτρέπει στους χρήστες να εκτελούν υπολογισμούς μεταφοράς φορτίου χωρίς καμία λήψη ή εγκατάσταση!
βιβλιογραφικές αναφορές:
- ο, Π.Μ.. (2007). Αρχές Μηχανικής του Ιδρύματος (7ου Έκδοση). Παγκόσμια Μηχανική
- Rajapakse, Ρ. (2016). Σχεδιασμός και κατασκευή κανόνα σωρού του αντίχειρα (2nd Έκδοση). Elsevier Inc..
- Τομλίνσον, Μ.Γ.. (2004). Πρακτική σχεδιασμού και κατασκευής σωρών (4ου Έκδοση). μι & FN Spon.
