Base Plate Design Example using EN 1993-1-8-2005, IM 1993-1-1-2005 und EN 1992-1-1-2004
Problemanweisung:
Determine whether the designed column-to-base plate connection is sufficient for a 100-kN compression load.
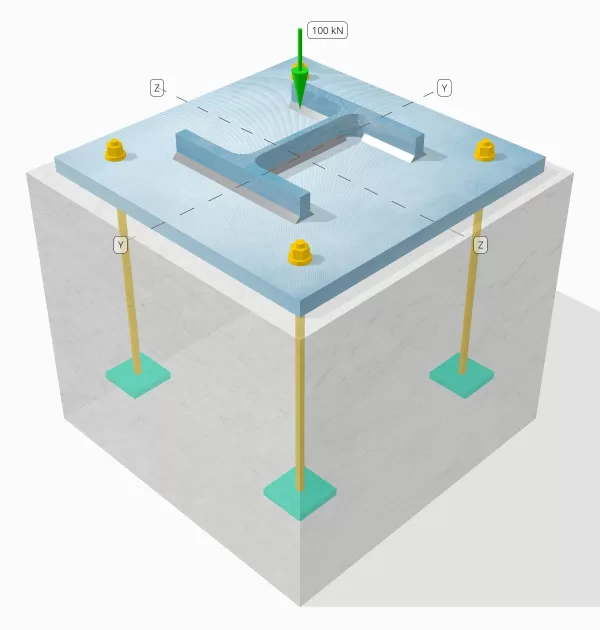
Gegebene Daten:
Spalte:
Spaltenabschnitt: HE 200 B.
Säulenbereich: 7808 mm2
Säulenmaterial: S235
Grundplatte:
Grundplattenabmessungen: 400 mmx 400 mm
Grundplattendicke: 20 mm
Grundplattenmaterial: S235
Grout:
Grout thickness: 20 mm
Beton:
Konkrete Abmessungen: 450 mmx 450 mm
Betondicke: 380 mm
Betonmaterial: C20/25
Schweißnähte:
Compression load transferred through welds only? NEIN
Schritt-für-Schritt-Berechnungen:
Prüfen #1: Berechnen Sie die Schweißkapazität
Since the compression load is not transferred through welds alone, a proper contact bearing surface is required to ensure that the load is transferred via bearing. Refer to IM 1090-2:2018 Klausel 6.8 for contact bearing preparation.
zusätzlich, use minimum weld size specified in Eurocode.
Prüfen #2: Calculate concrete bearing capacity and base plate yield capacity
The first step is to determine the design compressive strength of the joint, which depends on the geometry of the support (Beton) and the geometry of the loaded area (base plate).
We begin by calculating the alpha factor, which accounts for the diffusion of the concentrated force within the foundation.
Gemäß IM 1992-1-1:2004, Klausel 6.7, the alpha coefficient is the ratio of the loaded area to the maximum distribution area, which has a similar shape to the loaded area.
We will use the equation from Teil 6.1 of Multi-Storey Steel Buildings Part 5 durch Arcelor Mittal, Peiner Träger, und Corus to calculate the alpha factor.
\(
\alpha = \min \left(
1 + \frac{t_{\Text{conc}}}{\max(L_{\Text{bp}}, B_{\Text{bp}})},
1 + 2 \links( \frac{e_h}{L_{\Text{bp}}} \richtig),
1 + 2 \links( \frac{e_b}{B_{\Text{bp}}} \richtig),
3
\richtig)
\)
\(
\alpha = \min \left(
1 + \frac{380 \, \Text{mm}}{\max(400 \, \Text{mm}, 400 \, \Text{mm})},
1 + 2 \links( \frac{25 \, \Text{mm}}{400 \, \Text{mm}} \richtig),
1 + 2 \links( \frac{25 \, \Text{mm}}{400 \, \Text{mm}} \richtig),
3
\richtig)
\)
\(
\alpha = 1.125
\)
wo,
\(
e_h = \frac{L_{\Text{conc}} – L_{\Text{bp}}}{2} = frac{450 \, \Text{mm} – 400 \, \Text{mm}}{2} = 25 \, \Text{mm}
\)
\(
e_b = \frac{B_{\Text{conc}} – B_{\Text{bp}}}{2} = frac{450 \, \Text{mm} – 400 \, \Text{mm}}{2} = 25 \, \Text{mm}
\)
Once the geometry is defined, we will then determine the compressive strength of the concrete using IM 1992-1-1:2004, Gl. 3.15.
\(
f_{CD} = frac{\= Abstand des Abschnitts, in dem die Scherung berücksichtigt wird, zur Fläche des nächsten Auflagers{cc} f_{ck}}{\gamma_C} = frac{1 \mal 20 \, \Text{MPa}}{1.5} = 13.333 \, \Text{MPa}
\)
Als nächstes, we assume a value for the beta coefficient. Since grout is present, beta value can be 2/3. We will calculate the design bearing strength of the joint using the combined formulas from IM 1993-1-8:2005 Gl. 6.6, und IM 1992-1-1:2004 Gl. 6.63.
\(
f_{jd} = \beta \alpha f_{CD} = 0.66667 \mal 1.125 \mal 13.333 \, \Text{MPa} = 10 \, \Text{MPa}
\)
The second part involves calculating the base plate yield capacity.
Since we already have the design bearing strength of the connection, we will use this to determine the smallest cantilever distance of the base plate that experiences the full bearing load. We will refer to the SCI P358 example on page 243 und IM 1993-1-1:2005 Klausel 6.2.5.
\(
c = t_{\Text{bp}} \sqrt{\frac{f_{y_{\Text{bp}}}}{3 f_{jd} \Um es zu berechnen{M0}}} = 20 \, \Text{mm} \mal sqrt{\frac{225 \, \Text{MPa}}{3 \mal 10 \, \Text{MPa} \mal 1}} = 54.772 \, \Text{mm}
\)
We will use this dimension to calculate the effective area of the base plate. The ‘c’ dimension we calculated may overlap or not overlap near the flange. If it overlaps, we will assume the section to be a rectangular section. If it does not overlap, we will take the shape of the column.
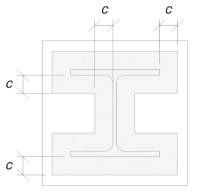
Without overlap
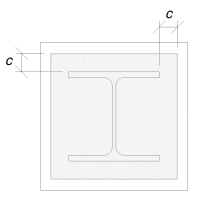
With overlap
We determined that the ‘c’ dimension does not overlap. Deshalb, mit SCI P358 pg. 243, the effective area is:
\(
A_e = 4c^2 + P_{\Text{col}}c + EIN_{\Text{col}} = 4 \times 54.772^2 \, \Text{mm}^ 2 + 1182 \, \Text{mm} \mal 54.772 \, \Text{mm} + 7808 \, \Text{mm}^2 = 84549 \, \Text{mm}^ 2
\)
It is important to note that the effective area should not be less than the base plate area.
Schließlich, wir werden verwenden IM 1993-1-8:2005 Gl. 6.6, und EN 1992-1-1:2004, Gl. 6.63 to calculate the design bearing resistance of the base plate connection.
\(
N_{Rd} = left( \Min.(A_e, A_0) \richtig) f_{jd} = left( \Min.(84549 \, \Text{mm}^ 2, 160000 \, \Text{mm}^ 2) \richtig) \mal 10 \, \Text{MPa} = 845.49 \, \Text{kN}
\)
Schon seit 845.49 kN > 100 kN, the design is ausreichend!
Entwurfszusammenfassung
Die Skyciv-Basisplattentwurfsoftware kann automatisch einen schrittweisen Berechnungsbericht für dieses Entwurfsbeispiel erstellen. Es enthält auch eine Zusammenfassung der durchgeführten Schecks und deren resultierenden Verhältnisse, Die Informationen auf einen Blick leicht zu verstehen machen. Im Folgenden finden Sie eine Stichprobenzusammenfassungstabelle, Welches ist im Bericht enthalten.
SKYCIV -Beispielbericht
Klicke hier to download a sample report.
Basisplattensoftware kaufen
Kaufen Sie die Vollversion des Basisplatten -Designmoduls selbst ohne andere Skyciv -Module selbst. Auf diese Weise erhalten Sie einen vollständigen Satz von Ergebnissen für die Basisplattendesign, Einbeziehung detaillierter Berichte und mehr Funktionen.
