Basisplatten -Design -Beispiel mit eN 1993-1-8:2005, IM 1993-1-1:2005, IM 1992-1-1:2004, und EN 1992-4:2018.
Problemanweisung:
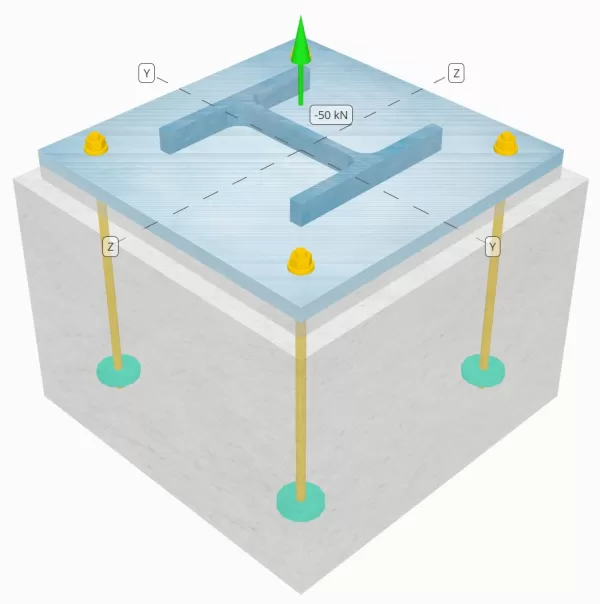
Bestimmen Sie, ob die konstruierte Säule-zu-Base-Plattenverbindung für eine Spannungsbelastung von 50 km ausreicht.
Gegebene Daten:
Spalte:
Spaltenabschnitt: HE 240 B.
Säulenbereich: 10600 mm2
Säulenmaterial: S235
Grundplatte:
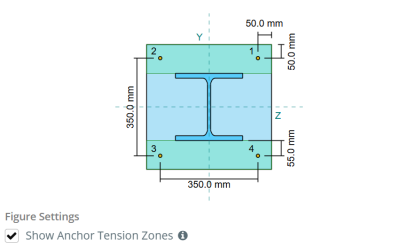
Grundplattenabmessungen: 450 mmx 450 mm
Grundplattendicke: 20 mm
Grundplattenmaterial: S235
Fugenmörtel:
Fugenmörtel Dicke: 20 mm
Beton:
Konkrete Abmessungen: 500 mmx 500 mm
Betondicke: 350 mm
Betonmaterial: C25/30
Geknackt oder ungekrönt: Geknackt
Anker:
Ankerdurchmesser: 12 mm
Effektive Einbettungslänge: 300.0 mm
Eingebetteter Plattendurchmesser: 60 mm
Dicke eingebetteter Platten: 10 mm
Ankermaterial: 8.8
Andere Informationen:
- Nicht-Counter-sunk-Anker.
- Anker mit geschnittenen Fäden.
Schweißnähte:
Schweißtyp: FPBW
Füllmetallklassifizierung: E35
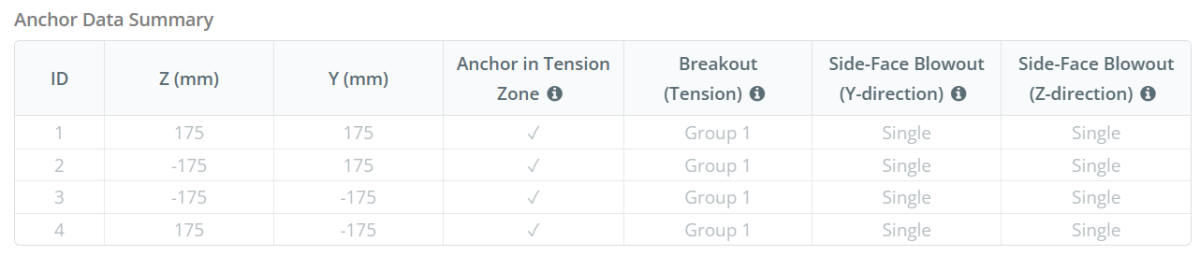
Ankerdaten (von Skyciv -Taschenrechner):
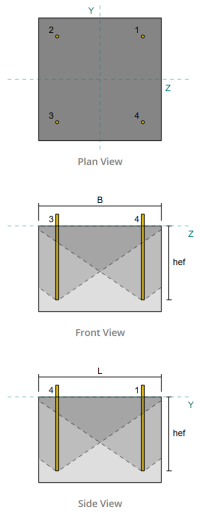
Definitionen:
Anchor Tension Zone:
In dem SkyCiv Basisplatten-Design-Software, Nur Anker innerhalb der Ankerspannungszone werden als wirksam angesehen, um die Häufigkeit zu widerstehen. Diese Zone enthält typischerweise Bereiche in der Nähe der Spaltenflansche oder des Netzes. Anker außerhalb dieser Zone tragen nicht zum Spannungswiderstand bei und sind von den Anhebungsberechnungen ausgeschlossen.
Die Annahme vereinfacht die Grundplattenanalyse, indem sie sich annähert, wie sich die Erhöhungskraft durch die Platte ausbreitet.
Ankergruppen:
Mit der SkyCiv Basisplatten-Design-Software Enthält eine intuitive Funktion, die identifiziert, welche Anker Teil einer Ankergruppe für die Bewertung sind Betonausbruch und Beton-Seitenflächen-Blowout Fehler.
Ein Ankergruppe besteht aus mehreren Ankern mit ähnlichen effektiven Einbettungstiefen und Abstand, und sind nah genug, dass ihre Projizierte Widerstandsbereiche überlappen sich. Wenn Anker gruppiert sind, Ihre Kapazitäten werden kombiniert, um der Gesamtspannungskraft zu widerstehen, die der Gruppe angewendet wird.
Anker, die die Gruppierungskriterien nicht erfüllen, werden als behandelt einzelne Anker. In diesem Fall, Nur die Spannungskraft am individuellen Anker wird gegen seinen eigenen wirksamen Widerstandsbereich überprüft.
Schritt-für-Schritt-Berechnungen:
Prüfen #1: Berechnen Sie die Schweißkapazität
From the given information, the weld used in this design example is a Full Penetration Butt Weld (FPBW). We will calculate the base metal capacities of the column and the base plate to determine the weld resistance. Um dies zu tun, we first need to calculate the Gesamtschweißlänge on the column and obtain the weld stress.
\(
F_{w,Ed} = frac{N_x}{2 b_f t_f + \links( d_{col} – 2 t_f – 2 r_{col} \richtig) t_w}
\)
\(
F_{w,Ed} = frac{50 \, \Text{kN}}{2 \mal 240 \, \Text{mm} \mal 17 \, \Text{mm} + \links( 240 \, \Text{mm} – 2 \mal 17 \, \Text{mm} – 2 \mal 21 \, \Text{mm} \richtig) \mal 10 \, \Text{mm}} = 5.102 \, \Text{MPa}
\)
Als nächstes, Wir bestimmen die tensile strength of the weaker material between the column and the base plate.
\(
f_y = \min \left( f_{j,\Text{col}}, f_{j,\Text{bp}} \richtig) = min links( 225 \, \Text{MPa}, 225 \, \Text{MPa} \richtig) = 225 \, \Text{MPa}
\)
Wir verwenden dann IM 1993-1-8:2005 Klausel 4.7.1 und IM 1993-1-1:2005 Gl. 6.6 to calculate the FPBW design weld resistance.
\(
F_{w,Rd3} = frac{f_y}{\Um es zu berechnen{M0}} = frac{225 \, \Text{MPa}}{1} = 225 \, \Text{MPa}
\)
Schon seit 5.102 MPa < 225 MPa, Die Schweißkapazität ist ausreichend.
Prüfen #2: Berechnen Sie die Kapazität der Grundplattenflexus aufgrund der Spannungsbelastung
Zur Berechnung der base plate flexural capacity against tension load, wir werden verwenden yield line patterns such as circular patterns and non-circular patterns. Dann, we determine the governing capacity, assuming no prying forces, by comparing the plate’s yielding strength with the tensile resistance of the anchor bolts.
Anfangen, we compute the required Abmessungen based on the given bolt layout. Beziehen sich auf IM 1992-1-8:2005 Tabelle 6.2 for guidance.
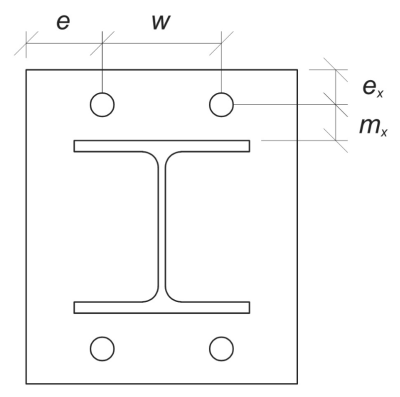
\(
m_x = \frac{S_ – d_{col}}{2} = frac{350 \, \Text{mm} – 240 \, \Text{mm}}{2} = 55 \, \Text{mm}
\)
\(
w = s_z \left( N_{ein,\Text{Seite}} – 1 \richtig) = 350 \, \Text{mm} \mal links( 2 – 1 \richtig) = 350 \, \Text{mm}
\)
\(
e_x = \frac{L_{bp} – S_}{2} = frac{450 \, \Text{mm} – 350 \, \Text{mm}}{2} = 50 \, \Text{mm}
\)
\(
e = frac{B_{bp} – w}{2} = frac{450 \, \Text{mm} – 350 \, \Text{mm}}{2} = 50 \, \Text{mm}
\)
\(
b_p = B_{bp} = 450 \, \Text{mm}
\)
Let us also compute the anchor edge distance on the base plate, which is limited by the \( m_x \) dimension per
\(
n = \min \left( e_x, 1.25 m_x \right) = min links( 50 \, \Text{mm}, 1.25 \mal 55 \, \Text{mm} \richtig) = 50 \, \Text{mm}
\)
Dann, we calculate the effective lengths ofthe following circular patterns (beziehen auf SCI P398 Table 5.3).
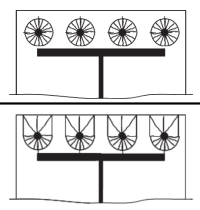
Circular pattern 1:
\(
l_{eff,cp1} = n_{ein,\Text{Seite}} \pi m_x = 2 \times \pi \times 55 \, \Text{mm} = 345.58 \, \Text{mm}
\)
Circular pattern 2:
\(
l_{eff,cp2} = left( \frac{N_{ein,\Text{Seite}}}{2} \richtig) (\pi m_x + 2 e_x) = left( \frac{2}{2} \richtig) \mal (\pi \times 55 \, \Text{mm} + 2 \mal 50 \, \Text{mm}) = 272.79 \, \Text{mm}
\)
Governing circular pattern effektive Länge:
\(
l_{eff,cp} = min (l_{eff,cp1}, l_{eff,cp2}) = min (345.58 \, \Text{mm}, 272.79 \, \Text{mm}) = 272.79 \, \Text{mm}
\)
Jetzt, we calculate the effective lengths of the following non-circular patterns (beziehen auf SCI P398 Table 5.3)
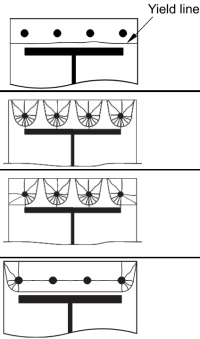
Non-circular pattern 1:
\(
l_{eff,nc1} = frac{b_p}{2} = frac{450 \, \Text{mm}}{2} = 225 \, \Text{mm}
\)
Non-circular pattern 2:
\(
l_{eff,nc2} = left( \frac{N_{ein,\Text{Seite}}}{2} \richtig) (4 m_x + 1.25 e_x) = left( \frac{2}{2} \richtig) \mal (4 \mal 55 \, \Text{mm} + 1.25 \mal 50 \, \Text{mm}) = 282.5 \, \Text{mm}
\)
Non-circular pattern 3:
\(
l_{eff,nc3} = 2 m_x + 0.625 e_x + e = 2 \mal 55 \, \Text{mm} + 0.625 \mal 50 \, \Text{mm} + 50 \, \Text{mm} = 191.25 \, \Text{mm}
\)
Non-circular pattern 4:
\(
l_{eff,nc4} = 2 m_x + 0.625 e_x + \frac{(N_{ein,\Text{Seite}} – 1) s_z}{2} = 2 \mal 55 \, \Text{mm} + 0.625 \mal 50 \, \Text{mm} + \frac{(2 – 1) \mal 350 \, \Text{mm}}{2} = 316.25 \, \Text{mm}
\)
Governing non-circular pattern effektive Länge:
\(
l_{eff,nc} = min (l_{eff,nc1}, l_{eff,nc2}, l_{eff,nc3}, l_{eff,nc4}) = min (225 \, \Text{mm}, 282.5 \, \Text{mm}, 191.25 \, \Text{mm}, 316.25 \, \Text{mm}) = 191.25 \, \Text{mm}
\)
Dann, we determine the lesser value between the effective lengths of the circular and non-circular patterns.
\(
l_{eff,1} = min (l_{eff,cp}, l_{eff,nc}) = min (272.79 \, \Text{mm}, 191.25 \, \Text{mm}) = 191.25 \, \Text{mm}
\)
Jetzt, we use this computed effective length to calculate its flexural yielding resistance. Gemäß IM 1993-1-8:2005 Tabelle 6.2, the plate moment resistance for failure Mode 1 ist:
\(
M_{pl,1,Rd} = frac{0.25 l_{eff,1} (t_{bp})^2 f_{und _bp}}{\Um es zu berechnen{M0}} = frac{0.25 \mal 191.25 \, \Text{mm} \mal (20 \, \Text{mm})^2 mal 225 \, \Text{MPa}}{1} = 4303.1 \, \Text{kN} \CDOT Text{mm}
\)
Assuming no prying, we use EN 1993-1-8:2005 Tabelle 6.2 to determine the Design resistance of the base plate for failure Modes 1 und 2.
\(
F_{T.,1,Rd} = frac{2 M_{pl,1,Rd}}{m_x} = frac{2 \mal 4303.1 \, \Text{kN} \CDOT Text{mm}}{55 \, \Text{mm}} = 156.48 \, \Text{kN}
\)
Dann, we calculate the tensile resistance of the anchor rod using IM 1992-4:2018 Klausel 7.2.1.3. This will be further detailed in the succeeding anchor checks.
\(
F_{t,Rd} = frac{c k_2 F_{U _anc} Als}{\Um es zu berechnen{M2,anchor}} = frac{0.85 \mal 0.9 \mal 800 \, \Text{MPa} \mal 113.1 \, \Text{mm}^ 2}{1.25} = 55.372 \, \Text{kN}
\)
We will then use the resistance per anchor rod to calculate the design resistance of the base plate under failure Modus 3, which is the total bolt failure.
\(
F_{T.,3,Rd} = n_{ein,Seite} F_{t,Rd} = 2 \mal 55.372 \, \Text{kN} = 110.74 \, \Text{kN}
\)
Schließlich, we determine the governing resistance value among the failure modes.
\(
F_{T.,Rd} = min (F_{T.,1,Rd}, F_{T.,3,Rd}) = min (156.48 \, \Text{kN}, 110.74 \, \Text{kN}) = 110.74 \, \Text{kN}
\)
Calculating the tension load per flange, wir haben:
\(
F_{T.,Ed} = frac{N_x}{2} = frac{50 \, \Text{kN}}{2} = 25 \, \Text{kN}
\)
Schon seit 25 kN < 110.74 kN, Die Grundkapazität der Grundplattenbiegung ist ausreichend.
Prüfen #3: Berechnen Sie die Ankerstange Zugkapazität
We already know the value for the anchor rod tensile capacity, but let’s tackle it in more detail.
Zuerst, let’s calculate the tensile stress area of the anchor rod.
\(
A_s = frac{\Pi}{4} (d_{anc})^2 = Frac{\Pi}{4} \mal (12 \, \Text{mm})^2 = 113.1 \, \Text{mm}^ 2
\)
Dann, let’s apply the values for the \( c \) factor and the \( k_{2} \) Faktor. These values can be modified in the settings of the SkyCiv Base Plate Design software. Try free version here.
- \( c = 0.85 \) for anchors with cut threads
- \( k_{2} = 0.9\) for non-countersunk anchor
Jetzt, let’s use IM 1992-4:2018 Klausel 7.2.1.3 um die zu berechnen design resistance of anchor rod unter Spannung.
\(
N_{Rd,s} = frac{c k_2 F_{U _anc} Als}{\Um es zu berechnen{M2,anchor}} = frac{0.85 \mal 0.9 \mal 800 \, \Text{MPa} \mal 113.1 \, \Text{mm}^ 2}{1.25} = 55.372 \, \Text{kN}
\)
Calculating the Spannungsbelastung pro Anker, wir haben:
\(
N_{Ed} = frac{N_x}{N_{ein,t}} = frac{50 \, \Text{kN}}{4} = 12.5 \, \Text{kN}
\)
Schon seit 12.5 kN < 55.372 kN, Die Ankerstange -Zugkapazität ist ausreichend.
Prüfen #4: Berechnen Sie die Betonausbruchkapazität in der Spannung
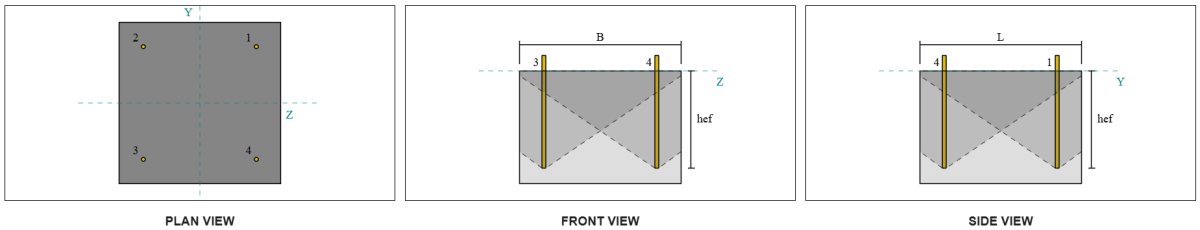
Vor der Berechnung der Ausbruchkapazität, Wir müssen zuerst feststellen, ob das Mitglied als qualifiziert ist enges Mitglied. Gemäß IM 1992-4:2008 Klausel 7.2.1.4(8), Das Mitglied erfüllt die Kriterien für ein enges Mitglied. Deshalb, ein verändert Effektive Einbettungslänge muss in den Ausbruchkapazitätsberechnungen verwendet werden. Diese Anpassung beeinflusst auch die charakteristischer Abstand und charakteristische Kantenabstand, das muss entsprechend geändert werden.
Basierend auf den schmalen Mitgliedskriterien, bleibt die Modifizierte Werte Für die Ankergruppe sind wie folgt:
- modifizierte effektive Einbettungslänge, \( H'_{ef} = 100 mm \)
- modifizierter charakteristischer Abstand, \( S'_{Einstellungen für Biege-Torsionsknicken} = 300 mm\)
- modifizierter charakteristischer Kantenentfernung, \( C'_{Einstellungen für Biege-Torsionsknicken} = 150 mm\)
Verwenden von IM 1992-4:2018 Gl. 7.3, wir berechnen die Referenz projizierter Betonkegelbereich für einen einzelnen Anker.
\(
A0_{c,N.} = s’_{Einstellungen für Biege-Torsionsknicken,G1} S'_{Einstellungen für Biege-Torsionsknicken,G1} = 350 \, \Text{mm} \mal 350 \, \Text{mm} = 122500 \, \Text{mm}^ 2
\)
Ähnlich, wir berechnen die Tatsächlicher projizierter Betonkegelbereich der Ankergruppe.
\(
EIN_{Nc} = L_{Nc} B_{Nc} = 500 \, \Text{mm} \mal 500 \, \Text{mm} = 250000 \, \Text{mm}^ 2
\)
Wo,
\(
L_{Nc} = min links( c_{links,G1}, C'_{Einstellungen für Biege-Torsionsknicken,G1} \richtig)
+ \links( \min links( S_{Summe,mit,G1}, S'_{Einstellungen für Biege-Torsionsknicken,G1} \links( N_{mit,G1} – 1 \richtig) \richtig) \richtig)
+ \min links( c_{richtig,G1}, C'_{Einstellungen für Biege-Torsionsknicken,G1} \richtig)
\)
\(
L_{Nc} = min links( 75 \, \Text{mm}, 175 \, \Text{mm} \richtig)
+ \links( \min links( 350 \, \Text{mm}, 350 \, \Text{mm} \mal (2 – 1) \richtig) \richtig)
+ \min links( 75 \, \Text{mm}, 175 \, \Text{mm} \richtig)
\)
\(
L_{Nc} = 500 \, \Text{mm}
\)
\(
B_{Nc} = min links( c_{oben,G1}, C'_{Einstellungen für Biege-Torsionsknicken,G1} \richtig)
+ \links( \min links( S_{Summe,j,G1}, S'_{Einstellungen für Biege-Torsionsknicken,G1} \links( N_{j,G1} – 1 \richtig) \richtig) \richtig)
+ \min links( c_{Unterseite,G1}, C'_{Einstellungen für Biege-Torsionsknicken,G1} \richtig)
\)
\(
B_{Nc} = min links( 75 \, \Text{mm}, 175 \, \Text{mm} \richtig)
+ \links( \min links( 350 \, \Text{mm}, 350 \, \Text{mm} \mal (2 – 1) \richtig) \richtig)
+ \min links( 75 \, \Text{mm}, 175 \, \Text{mm} \richtig)
\)
\(
B_{Nc} = 500 \, \Text{mm}
\)
Als nächstes, Wir bewerten die charakteristische Stärke eines einzelnen Ankers verwenden IM 1992-4:2018 Gl. 7.2
\(
N0_{Rk,c} = k_1 sqrt{\frac{f_{ck}}{\Text{MPa}}} \links( \frac{H'_{ef,G1}}{\Text{mm}} \richtig)^{1.5} N.
\)
\(
N0_{Rk,c} = 8.9 \mal sqrt{\frac{25 \, \Text{MPa}}{1 \, \Text{MPa}}} \mal links( \frac{116.67 \, \Text{mm}}{1 \, \Text{mm}} \richtig)^{1.5} \mal 0.001 \, \Text{kN} = 56.076 \, \Text{kN}
\)
Wo,
- \(k_{1} = 8.9\) für einbetonierte Anker
Jetzt, Wir bewerten die Auswirkungen der Geometrie, indem wir die erforderlichen Berechnung berechnen Parameter für Breakout -Widerstand.
Der kürzeste Randabstand der Ankergruppe wird als bestimmt als:
\(
c_{Min.,N.} = min links( c_{links,G1}, c_{richtig,G1}, c_{oben,G1}, c_{Unterseite,G1} \richtig)
= min links( 87.5 \, \Text{mm}, 87.5 \, \Text{mm}, 150 \, \Text{mm}, 150 \, \Text{mm} \richtig)
= 87.5 \, \Text{mm}
\)
Gemäß IM 1992-4:2018 Gl. 7.4, Der Wert für den Parameter zur Verteilung der Spannung in Beton ist:
\(
\Psi_{s,N.} = min links( 0.7 + 0.3 \links( \frac{c_{Min.,N.}}{C'_{Einstellungen für Biege-Torsionsknicken,G1}} \richtig), 1.0 \richtig)
= min links( 0.7 + 0.3 \mal links( \frac{75 \, \Text{mm}}{175 \, \Text{mm}} \richtig), 1 \richtig)
= 0.82857
\)
Mit der Shell Spalling -Effekt wird zur Verwendung berücksichtigt IM 1992-4:2018 Gl. 7.5, geben:
\(
\Psi_{Ausbruchkegelbereich für Einzeldübel nicht durch Kanten beeinflusst,N.} = min links( 0.5 + \frac{H'_{ef,G1}}{\Text{mm} \, / \, 200}, 1.0 \richtig)
= min links( 0.5 + \frac{116.67 \, \Text{mm}}{1 \, \Text{mm} \, / \, 200}, 1 \richtig)
= 1
\)
Zusätzlich, beide Exzentrizitätsfaktor und das Druckeinflussfaktor werden als:
\(
\Psi_{ec,N.} = 1
\)
\(
\Psi_{M.,N.} = 1
\)
Wir kombinieren dann all diese Faktoren und bewerben uns AS 5216:2021 Gleichung 6.2.3.1 Um die zu bewerten Konstruktionskegelausbruchwiderstand entwerfen Für die Ankergruppe:
\(
N_{Rd,c} = frac{N0_{Rk,c} \links( \frac{EIN_{Nc}}{A0_{c,N.}} \richtig) \Psi_{s,N.} \Psi_{Ausbruchkegelbereich für Einzeldübel nicht durch Kanten beeinflusst,N.} \Psi_{ec,N.} \Psi_{M.,N.}}{\Um es zu berechnen{Mc}}
\)
\(
N_{Rd,c} = frac{56.076 \, \Text{kN} \mal links( \frac{250000 \, \Text{mm}^ 2}{122500 \, \Text{mm}^ 2} \richtig) \mal 0.82857 \mal 1 \mal 1 \mal 1}{1.5} = 63.215 \, \Text{kN}
\)
Mit der Gesamtspannungsbelastung Auf der Ankergruppe wird berechnet, indem die Spannungsbelastung pro Anker mit der Anzahl der Anker multipliziert wird:
\(
N_{Fa} = left( \frac{N_x}{N_{ein,t}} \richtig) N_{ein,G1} = left( \frac{50 \, \Text{kN}}{4} \richtig) \mal 4 = 50 \, \Text{kN}
\)
Schon seit 50 kN < 63.215 kN Die Betonausbruchkapazität ist ausreichend.
Prüfen #5: Berechnen Sie die Ankerauszugskapazität
Mit der Ausziehkapazität eines Ankers wird durch den Widerstand an seinem eingebetteten Ende bestimmt. Anwenden seismischer Lasten, wir berechnen die bearing area of the embedded plate, which is the net area after subtracting the area occupied by the anchor rod.
Zuerst, Wir berechnen die maximale Ankerkopfdimension, die für den Auszugswiderstand wirksam ist, gemäß IM 1992-4:2018 Klausel 7.2.1.5 Hinweis.
\(
d_{h,\Text{max}} = min links( = Abstand des Abschnitts, in dem die Scherung berücksichtigt wird, zur Fläche des nächsten Auflagers{\Text{einbetten _plate}}, 6 \links( t_{\Text{einbetten _plate}} \richtig) + d_{\Text{anc}} \richtig)
= min links( 60 \, \Text{mm}, 6 \mal (10 \, \Text{mm}) + 12 \, \Text{mm} \richtig)
= 60 \, \Text{mm}
\)
Als nächstes, we calculate the net bearing area of the circular embedded plate using:
\(
EIN_{brg} = frac{\Pi}{4} \links( \links( d_{h,\Text{max}} \richtig)^ 2 – \links( d_{\Text{anc}} \richtig)^2 rechts)
\)
\(
EIN_{brg} = frac{\Pi}{4} \mal links( \links( 60 \, \Text{mm} \richtig)^ 2 – \links( 12 \, \Text{mm} \richtig)^2 rechts) = 2714.3 \, \Text{mm}^ 2
\)
Wir berechnen dann die design concrete pullout resistance of cast-in anchor in tension using IM 1992-4:2018 Klausel 7.2.1.5:
\(
N_{Rd,s} = frac{k_2 A_{brg} f_{ck}}{\Um es zu berechnen{Mp}}
= frac{7.5 \mal 2714.3 \, \Text{mm}^2 mal 25 \, \Text{MPa}}{1.5}
= 339.29 \, \Text{kN}
\)
Erinnern Sie sich an die zuvor berechneten Spannungsbelastung pro Anker:
\(
N_{Ed} = frac{N_x}{N_{ein,t}} = frac{50 \, \Text{kN}}{4} = 12.5 \, \Text{kN}
\)
Schon seit 12.5 kN < 339.29 kN, Die Ankerauszugskapazität ist ausreichend.
Prüfen #6: Berechnen Sie die Blowout-Kapazität der Seitengesicht in der y-Richtung
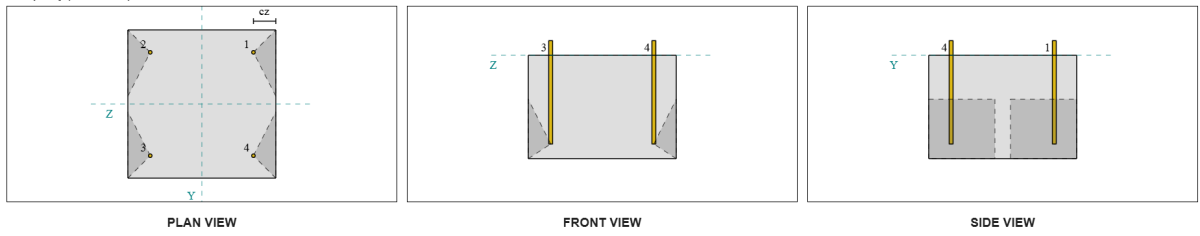
Let’s consider Anchor ID #3. Wir beginnen mit der Berechnung des Kantenabstands zur Fehlerkante.
\(
c_{mit,\Text{Min.}} = min links( c_{\Text{links,s3}}, c_{\Text{richtig,s3}} \richtig)
= min links( 75 \, \Text{mm}, 425 \, \Text{mm} \richtig)
= 75 \, \Text{mm}
\)
Als nächstes, Wir bestimmen den Randabstand zum orthogonale Kante.
\(
c_{j,\Text{Min.}} = min links( c_{\Text{oben,s3}}, c_{\Text{Unterseite,s3}} \richtig)
= min links( 425 \, \Text{mm}, 75 \, \Text{mm} \richtig)
= 75 \, \Text{mm}
\)
Verwenden von IM 1992-4:2018 Gl. 7.27, Berechnen wir die Referenz projizierter Bereich eines einzelnen Befestigers.
\(
A0_{c,NB} = left( 4 c_{mit,\Text{Min.}} \richtig)^ 2
= left( 4 \mal 75 \, \Text{mm} \richtig)^ 2
= 90000 \, \Text{mm}^ 2
\)
Da wir die Kapazität der Ankergruppe überprüfen, Lass uns das bekommen Tatsächlicher projizierter Bereich der Ankergruppe verwenden IM 1992-4:2018 Gl. 7.27.
\(
EIN_{Nc} = B_{c,NB} Um es zu berechnen{c,NB} = 225 \, \Text{mm} \mal 200 \, \Text{mm} = 45000 \, \Text{mm}^ 2
\)
Wo,
\(
B_{c,NB} = 2 c_{mit,\Text{Min.}} + \min links( 2 c_{mit,\Text{Min.}}, c_{j,\Text{Min.}} \richtig)
= 2 \mal 75 \, \Text{mm} + \min links( 2 \mal 75 \, \Text{mm}, 75 \, \Text{mm} \richtig)
= 225 \, \Text{mm}
\)
\(
Um es zu berechnen{c,NB} = 2 c_{mit,\Text{Min.}} + \links( \min links( t_{\Text{konz}} – h_{\Text{ef}}, 2 c_{mit,\Text{Min.}} \richtig) \richtig)
= 2 \mal 75 \, \Text{mm} + \links( \min links( 350 \, \Text{mm} – 300 \, \Text{mm}, 2 \mal 75 \, \Text{mm} \richtig) \richtig)
= 200 \, \Text{mm}
\)
In der Berechnung der charakteristische konkrete Ausbruchstärke eines individuellen Ankers, wir werden verwenden IM 1992-4:2018 Gl. 7.26.
\(
N0_{Rk,cb} = k_5 links( \frac{c_{mit,\Text{Min.}}}{\Text{mm}} \richtig)
\links( \sqrt{\frac{EIN_{\Text{brg}}}{\Text{mm}^ 2}} \richtig)
\links( \sqrt{\frac{f_{ck}}{\Text{MPa}}} \richtig) N.
\)
\(
N0_{Rk,cb} = 8.7 \mal links( \frac{75 \, \Text{mm}}{1 \, \Text{mm}} \richtig)
\mal links( \sqrt{\frac{2714.3 \, \Text{mm}^ 2}{1 \, \Text{mm}^ 2}} \richtig)
\mal links( \sqrt{\frac{25 \, \Text{MPa}}{1 \, \Text{MPa}}} \richtig)
\mal 0.001 \, \Text{kN}
\)
\(
N0_{Rk,cb} = 169.97 \, \Text{kN}
\)
Dann, Wir werden das bekommen Seitengesichtsparameter.
Der Parameter, der die Störung der Verteilung von Spannungen in Beton ausgeht, kann berechnet werden IM 1992-4:2018 Gl. 7.28.
\(
\Psi_{s,NB} = min links( 0.7 + 0.3 \links( \frac{c_{j,\Text{Min.}}}{2 c_{mit,\Text{Min.}}} \richtig), 1.0 \richtig)
= min links( 0.7 + 0.3 \mal links( \frac{75 \, \Text{mm}}{2 \mal 75 \, \Text{mm}} \richtig), 1 \richtig)
= 0.85
\)
Zusätzlich, the factors for group effect and factor the influence of eccentricity are as follows:
\(
\Psi_{G,NB} = 1
\)
\(
\Psi_{ec,N.} = 1
\)
Schließlich, in Bezug auf AS 5216:2021 Gl. 6.2.7 Für Kopf -Ankerstangen, bleibt die Konstruktion konstruiere Ausbruchwiderstand ist:
\(
N_{Rk,cb} = frac{N0_{Rk,cb} \links( \frac{EIN_{Nc}}{A0_{c,NB}} \richtig) \links( \Psi_{s,NB} \richtig) \links( \Psi_{G,NB} \richtig) \links( \Psi_{ec,N.} \richtig)}{\Um es zu berechnen{Mc}}
\)
\(
N_{Rk,cb} = frac{169.97 \, \Text{kN} \mal links( \frac{45000 \, \Text{mm}^ 2}{90000 \, \Text{mm}^ 2} \richtig) \mal links( 0.85 \richtig) \mal links( 1 \richtig) \mal links( 1 \richtig)}{1.5} = 48.159 \, \Text{kN}
\)
Recall Spannungsbelastung pro Anker:
\(
N_{Ed} = frac{N_x}{N_{ein,t}} = frac{50 \, \Text{kN}}{4} = 12.5 \, \Text{kN}
\)
Schon seit 12.5 kN < 48.159 kN, Der Beton-Seitenflächen-Blowout entlang der Y-Region ist ausreichend.
Any other Anchor ID number can also be used and will yield the same result, Da ist das Design symmetrisch.
Prüfen #7: Berechnen Sie die Blowout-Kapazität der Seitengesicht in der Z-Richtung
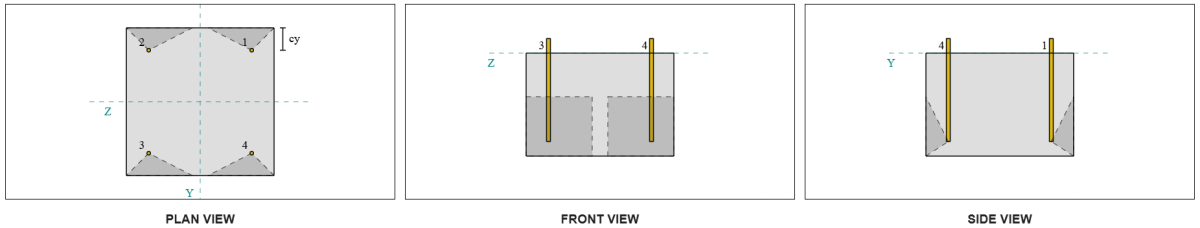
The same procedure is used in calculating the capacity for side-face blowout in Z-direction. Let’s consider Anchor ID #2 this time. Nochmal, we begin by calculating the edge distance to the Fehlerkante.
\(
c_{j,\Text{Min.}} = min links( c_{\Text{oben},s2}, c_{\Text{Unterseite},s2} \richtig)
= min links( 75 \, \Text{mm}, 425 \, \Text{mm} \richtig)
= 75 \, \Text{mm}
\)
Als nächstes, Wir bestimmen den Randabstand zum orthogonale Kante.
\(
c_{mit,\Text{Min.}} = min links( c_{\Text{links},s2}, c_{\Text{richtig},s2} \richtig)
= min links( 75 \, \Text{mm}, 425 \, \Text{mm} \richtig)
= 75 \, \Text{mm}
\)
Verwenden von IM 1992-4:2018 Gl. 7.27, Berechnen wir die Referenz projizierter Bereich eines einzelnen Befestigers.
\(
A0_{c,NB} = left( 4 c_{j,\Text{Min.}} \richtig)^ 2
= left( 4 \mal 75 \, \Text{mm} \richtig)^ 2
= 90000 \, \Text{mm}^ 2
\)
Da wir die Kapazität der Ankergruppe überprüfen, Lass uns das bekommen Tatsächlicher projizierter Bereich der Ankergruppe verwenden IM 1992-4:2018 Gl. 7.27.
\(
EIN_{Nc} = B_{c,NB} Um es zu berechnen{c,NB}
= 225 \, \Text{mm} \mal 200 \, \Text{mm}
= 45000 \, \Text{mm}^ 2
\)
Wo,
\(
B_{c,NB} = 2 c_{j,\Text{Min.}} + \min links( 2 c_{j,\Text{Min.}}, c_{mit,\Text{Min.}} \richtig)
= 2 \mal 75 \, \Text{mm} + \min links( 2 \mal 75 \, \Text{mm}, 75 \, \Text{mm} \richtig)
= 225 \, \Text{mm}
\)
\(
Um es zu berechnen{c,NB} = 2 c_{j,\Text{Min.}} + \links( \min links( t_{\Text{konz}} – h_{\Text{ef}}, 2 c_{j,\Text{Min.}} \richtig) \richtig)
= 2 \mal 75 \, \Text{mm} + \links( \min links( 350 \, \Text{mm} – 300 \, \Text{mm}, 2 \mal 75 \, \Text{mm} \richtig) \richtig)
= 200 \, \Text{mm}
\)
In der Berechnung der charakteristische konkrete Ausbruchstärke eines individuellen Ankers, wir werden verwenden IM 1992-4:2018 Gl. 7.26.
\(
N0_{Rk,cb} = k_5 links( \frac{c_{j,\Text{Min.}}}{\Text{mm}} \richtig)
\sqrt{\links( \frac{EIN_{brg}}{\Text{mm}^ 2} \richtig)}
\sqrt{\links( \frac{f_{ck}}{\Text{MPa}} \richtig)} \, \Text{N.}
\)
\(
N0_{Rk,cb} = 8.7 \links( \frac{75 \, \Text{mm}}{1 \, \Text{mm}} \richtig)
\sqrt{\links( \frac{2714.3 \, \Text{mm}^ 2}{1 \, \Text{mm}^ 2} \richtig)}
\sqrt{\links( \frac{25 \, \Text{MPa}}{1 \, \Text{MPa}} \richtig)}
\Unterbau Erde 0.001 \, \Text{kN}
\)
\(
N0_{Rk,cb} = 169.97 \, \Text{kN}
\)
Dann, Wir werden das bekommen Seitengesichtsparameter.
Der Parameter, der die Störung der Verteilung von Spannungen in Beton ausgeht, kann berechnet werden IM 1992-4:2018 Gl. 7.28.
\(
\Psi_{s,NB} = min links( 0.7 + 0.3 \links( \frac{c_{mit,\Text{Min.}}}{2 c_{j,\Text{Min.}}} \richtig), 1.0 \richtig)
= min links( 0.7 + 0.3 \mal links( \frac{75 \, \Text{mm}}{2 \mal 75 \, \Text{mm}} \richtig), 1 \richtig)
= 0.85
\)
Zusätzlich, the factors for group effect and factor the influence of eccentricity are as follows:
\(
\Psi_{G,NB} = 1
\)
\(
\Psi_{ec,N.} = 1
\)
Schließlich, in Bezug auf AS 5216:2021 Gl. 6.2.7 Für Kopf -Ankerstangen, bleibt die Konstruktion konstruiere Ausbruchwiderstand ist:
Recall Spannungsbelastung pro Anker:
\(
N_{Ed} = frac{N_x}{N_{ein,t}} = frac{50 \, \Text{kN}}{4} = 12.5 \, \Text{kN}
\)
Schon seit 12.5 kN < 48.159 kN, the concrete side-face blowout along Z-direction is ausreichend.
Any other Anchor ID number can also be used and will yield the same result, Da ist das Design symmetrisch.
Entwurfszusammenfassung
Mit der Skyciv Base Plate Design Software kann automatisch einen Schritt-für-Schritt-Berechnungsbericht für dieses Entwurfsbeispiel erstellen. Es enthält auch eine Zusammenfassung der durchgeführten Schecks und deren resultierenden Verhältnisse, Die Informationen auf einen Blick leicht zu verstehen machen. Im Folgenden finden Sie eine Stichprobenzusammenfassungstabelle, Welches ist im Bericht enthalten.

SKYCIV -Beispielbericht
Klicke hier So laden Sie einen Beispielbericht herunter.
Basisplattensoftware kaufen
Kaufen Sie die Vollversion des Basisplatten -Designmoduls selbst ohne andere Skyciv -Module selbst. Auf diese Weise erhalten Sie einen vollständigen Satz von Ergebnissen für die Basisplattendesign, Einbeziehung detaillierter Berichte und mehr Funktionen.