Base Plate Design Example using CSA S16:19 and CSA A23.3:19
Probleemverklaring:
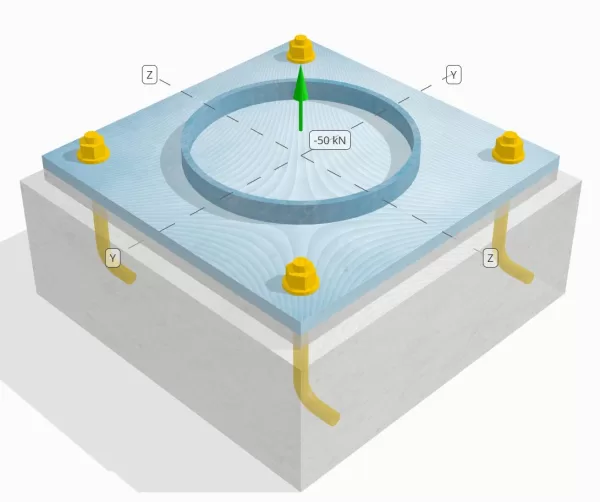
Bepaal of de ontworpen kolom-naar-base plaataansluiting voldoende is voor een spanningsbelasting van 50 knap.
Gegeven gegevens:
Kolom:
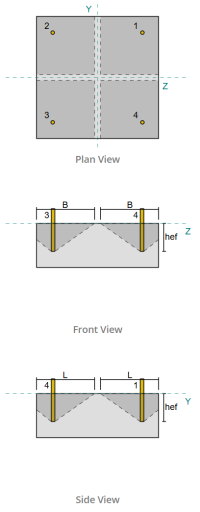
Kolomgedeelte: HS324X9.5
Kolomgebied: 9410 mm2
Kolommateriaal: 230G
Bodemplaat:
Baseplaat afmetingen: 500 mm x 500 mm
Basisplaatdikte: 20 mm
Basisplaatmateriaal: 230G
Vocht:
Vochtdikte: 20 mm
Beton:
Concrete dimensies: 550 mm x 550 mm
Betonnen dikte: 200 mm
Betonnen materiaal: 20.68 MPa
Gebarsten of ongescheurd: Gebarsten
Ankers:
Ankerdiameter: 19.1 mm
Effectieve inbeddingslengte: 130.0 mm
Hook length: 60mm
Anchor offset distance from face of column: 120.84 mm
Lassen:
Lastype: CJP
Vulmetaalclassificatie: E43XX
Ankergegevens (van Skyciv Calculator):

Definities:
Pad laden:
When a base plate is subjected to uplift (treksterkte) krachten, these forces are transferred to the anchor rods, which in turn induce bending moments in the base plate. The bending action can be visualized as cantilever bending occurring around the flanges or web of the column section, depending on where the anchors are positioned.
In de SkyCiv-software voor het ontwerpen van grondplaten, Alleen ankers in de ankerspanningszone worden als effectief beschouwd bij het weerstaan van opheffing. Deze zone bevat meestal gebieden in de buurt van de kolomflenzen of het web. In the case of a circular column, the anchor tension zone includes the entire area outside the column perimeter. Ankers buiten deze zone dragen niet bij aan spanningsweerstand en zijn uitgesloten van de Uplift -berekeningen.
To determine the effective area of the base plate that resists bending, een 45-degree dispersion is assumed from the centerline of each anchor rod toward the column face. This dispersion defines the effective weld length and helps establish the effective bending width van de plaat.
De veronderstelling vereenvoudigt de basisplaatanalyse door te benaderen hoe de opheffingskracht zich door de plaat verspreidt.
Ankergroepen:
De SkyCiv-software voor het ontwerpen van grondplaten Bevat een intuïtieve functie die identificeert welke ankers deel uitmaken van een ankergroep om te evalueren beton doorbraak en Concrete zij-gezichtsblaas mislukkingen.
Een ankergroep bestaat uit meerdere ankers met vergelijkbare effectieve inbeddingsdiepten en afstand, en zijn dichtbij genoeg dat hun geprojecteerde weerstandsgebieden overlappen elkaar. Wanneer ankers zijn gegroepeerd, Hun capaciteiten worden gecombineerd om de totale spanningskracht te weerstaan die op de groep wordt uitgeoefend.
Ankers die niet voldoen aan de groeperingscriteria worden behandeld als enkele ankers. In dit geval, Alleen de spanningskracht op het individuele anker wordt gecontroleerd tegen zijn eigen effectieve weerstandsgebied.
Stapsgewijze berekeningen:
Controleren #1: Lascapaciteit berekenen
Beginnen, we need to calculate the load per anchor and determine the effective weld length for each anchor. De effective weld length is based on a 45° dispersion line drawn from the center of the anchor to the face of the column. If this 45° line does not intersect the column, de tangent points are used instead. Bovendien, if the anchors are closely spaced, the effective weld length is reduced to avoid overlap. Uiteindelijk, the sum of all effective weld lengths must not exceed the actual weldable length available along the column circumference.
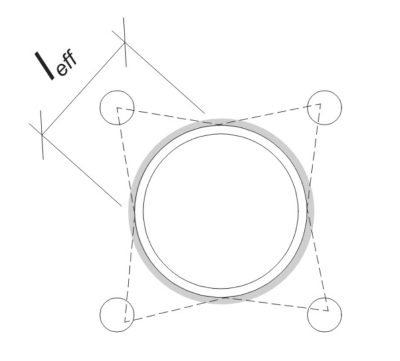
Let’s apply this to our example. Based on the given geometry, the 45° line from the anchor does not intersect the column. Als gevolg, the arc length between the tangent points is used instead. This arc length must also account for any adjacent anchors, with any overlapping portions subtracted to avoid double-counting. The calculated arc length is:
\(
l_{\tekst{boog}} = 254.47 \, \tekst{mm}
\)
This arc length calculation is fully automated in the SkyCiv Base Plate Design Software, but it can also be performed manually using trigonometric methods. You can try the free tool from this link.
Considering the available weldable length along the column’s circumference, the final effective weld length is:
\(
l_{\tekst{eff}} = min links( l_{\tekst{boog}}, \frac{\pi d_{\tekst{col}}}{N_{een,t}} \Rechtsaf) = min links( 254.47 \, \tekst{mm}, \frac{\pi \times 324 \, \tekst{mm}}{4} \Rechtsaf) = 254.47 \, \tekst{mm}
\)
De volgende, Laten we de load per anchor. For a given set of four (4) ankers, the load per anchor is:
\(
T_{u,\tekst{anker}} = frac{N_x}{N_{een,t}} = frac{50 \, \tekst{kN}}{4} = 12.5 \, \tekst{kN}
\)
Using the calculated effective weld length, we can now compute the required force per unit length acting on the weld.
\(
v_f = \frac{T_{u,\tekst{anker}}}{l_{\tekst{eff}}} = frac{12.5 \, \tekst{kN}}{254.47 \, \tekst{mm}} = 0.049122 \, \tekst{kN / mm}
\)
Nu, we refer to CSA S16:19 Clausule 13.13.3.1 om de factored resistance of the complete joint penetration (CJP) lassen. This requires the base metal resistance, expressed in force per unit length, for both the column and the base plate materials.
\(
v_{r,\tekst{bm}} = phi links( \min links( F_{j,\tekst{col}} t_{\tekst{col}}, F_{j,\tekst{bp}} t_{\tekst{bp}} \Rechtsaf) \Rechtsaf)
\)
\(
v_{r,\tekst{bm}} = 0.9 \keer links( \min links( 230 \, \tekst{MPa} \keer 9.53 \, \tekst{mm}, 230 \, \tekst{MPa} \keer 20 \, \tekst{mm} \Rechtsaf) \Rechtsaf) = 1.9727 \, \tekst{kN / mm}
\)
Sinds 0.049122 kN / mm < 1.9727 kN / mm, De lascapaciteit is voldoende.
Controleren #2: Bereken de buigcapaciteit van de basisplaat als gevolg van spanningsbelasting
Using the load per anchor and the offset distance from the center of the anchor to the face of the column, the moment applied to the base plate can be calculated using a cantilever assumption. For a circular column, the load eccentricity is determined by considering the sagitta of the welded arc, and can be calculated as follows:
\(
e_{\tekst{pipe}} = d_o + R_{\tekst{col}} \links( 1 – \cos links( \frac{l_{\tekst{eff}}}{2 R_{\tekst{col}}} \Rechtsaf) \Rechtsaf)
\)
\(
e_{\tekst{pipe}} = 120.84 \, \tekst{mm} + 162 \, \tekst{mm} \keer links( 1 – \cos links( \frac{254.47 \, \tekst{mm}}{2 \keer 162 \, \tekst{mm}} \Rechtsaf) \Rechtsaf) = 168.29 \, \tekst{mm}
\)
The induced moment is computed as:
\(
M_f = T_{u,\tekst{anker}} e_{\tekst{pipe}} = 12.5 \, \tekst{kN} \keer 168.29 \, \tekst{mm} = 2103.6 \, \tekst{kN} \cdot \text{mm}
\)
De volgende, we will determine the bending width of the base plate. Voor deze, we use the chord length corresponding to the effective weld arc.
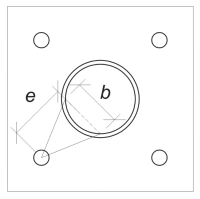
\(
\theta_{\tekst{rad}} = frac{l_{\tekst{eff}}}{0.5 d_{\tekst{col}}} = frac{254.47 \, \tekst{mm}}{0.5 \keer 324 \, \tekst{mm}} = 1.5708
\)
\(
b = d_{\tekst{col}} \links( \zonde links( \frac{\theta_{\tekst{rad}}}{2} \Rechtsaf) \Rechtsaf) = 324 \, \tekst{mm} \keer links( \zonde links( \frac{1.5708}{2} \Rechtsaf) \Rechtsaf) = 229.1 \, \tekst{mm}
\)
Uiteindelijk, we kunnen de berekenen factored flexural resistance of the base plate using CSA S16:19 Clausule 13.5.
\(
M_r = \phi F_{j,\tekst{bp}} Z_{\tekst{eff}} = 0.9 \keer 230 \, \tekst{MPa} \keer 22910 \, \tekst{mm}^3 = 4742.4 \, \tekst{kN} \cdot \text{mm}
\)
Waarbij,
\(
Z_{\tekst{eff}} = frac{b (t_{\tekst{bp}})^ 2}{4} = frac{229.1 \, \tekst{mm} \keer (20 \, \tekst{mm})^ 2}{4} = 22910 \, \tekst{mm}^3
\)
Sinds 2103.6 kN-mm < 4742.4 kN-mm, De buigplaten van de basisplaat is voldoende.
Controleren #3: Bereken de trekcapaciteit van de ankerstaaf
To evaluate the tensile capacity of the anchor rod, we refer to CSA A23.3:19 Clause D.6.1.2 and CSA S16:19 Clausule 25.3.2.1.
Eerste, We bepalen de specified tensile strength of the anchor steel. This is the lowest value permitted by CSA A23.3:19 Clause D.6.1.2.
\(
f_{\tekst{uta}} = min links( F_{u,\tekst{anc}}, 1.9 F_{j,\tekst{anc}}, 860 \Rechtsaf) = min links( 400 \, \tekst{MPa}, 1.9 \keer 248.2 \, \tekst{MPa}, 860.00 \, \tekst{MPa} \Rechtsaf) = 400 \, \tekst{MPa}
\)
De volgende, We bepalen de effective cross-sectional area of the anchor rod in tension using CAC Concrete Design Handbook, 3RD -editie, Tafel 12.3.
\(
EEN_{ik weet,N} = 215 \, \tekst{mm}^ 2
\)
With these values, We passen toe CSA A23.3:19 Eq. D.2 to compute the factored tensile resistance of the anchor rod.
\(
N_{\tekst{sar}} = A_{ik weet,N} \phi_s f_{\tekst{uta}} R = 215 \, \tekst{mm}^2 \times 0.85 \keer 400 \, \tekst{MPa} \keer 0.8 = 58.465 \, \tekst{kN}
\)
Bovendien, We evalueren de factored tensile resistance according to CSA S16:19 Clausule 25.3.2.1.
\(
T_r = \phi_{ar} 0.85 EEN_{ar} F_{u,\tekst{anc}} = 0.67 \keer 0.85 \keer 285.02 \, \tekst{mm}^2 \times 400 \, \tekst{MPa} = 64.912 \, \tekst{kN}
\)
After comparing the two, we identify that the factored resistance calculated using CSA A23.3:19 governs in this case.
Bedenk de eerder berekende spanningsbelasting per anker:
\(
N_{fa} = frac{N_x}{N_{een,t}} = frac{50 \, \tekst{kN}}{4} = 12.5 \, \tekst{kN}
\)
Sinds 12.5 kN < 58.465 kN, De trekcapaciteit van de ankerkam is voldoende.
Controleren #4: Bereken de betonuitbraakcapaciteit in spanning

Voordat u de breakout -capaciteit berekent, We moeten eerst bepalen of het lid in aanmerking komt als een smal lid. Volgens CSA A23.3:19 Clause D.6.2.3, the member does not meet the criteria for a narrow member. Daarom, the given Effectieve inbeddingslengte will be used in the calculations.
Gebruik makend van CSA A23.3:19 Eq. D.5, we berekenen de maximum projected concrete cone area voor één enkel anker, based on the effective embedment length.
\(
EEN_{Onthouden} = 9 (h_{ef,s1})➔⡔ Koop generieke tadalafil 9 \keer (130 \, \tekst{mm})➔⡔ Koop generieke tadalafil 152100 \, \tekst{mm}^ 2
\)
Evenzo, we use the effective embedment length to calculate the Werkelijk geprojecteerd betonnen kegelgebied of the single anchor.
\(
EEN_{Nc} = L_{Nc} B_{Nc} = 270 \, \tekst{mm} \keer 270 \, \tekst{mm} = 72900 \, \tekst{mm}^ 2
\)
Waarbij,
\(
L_{Nc} = links( \min links( c_{\tekst{links},s1}, 1.5 h_{ef,s1} \Rechtsaf) \Rechtsaf) + \links( \min links( c_{\tekst{Rechtsaf},s1}, 1.5 h_{ef,s1} \Rechtsaf) \Rechtsaf)
\)
\(
L_{Nc} = links( \min links( 475 \, \tekst{mm}, 1.5 \keer 130 \, \tekst{mm} \Rechtsaf) \Rechtsaf) + \links( \min links( 75 \, \tekst{mm}, 1.5 \keer 130 \, \tekst{mm} \Rechtsaf) \Rechtsaf)
\)
\(
L_{Nc} = 270 \, \tekst{mm}
\)
\(
B_{Nc} = links( \min links( c_{\tekst{top},s1}, 1.5 h_{ef,s1} \Rechtsaf) \Rechtsaf) + \links( \min links( c_{\tekst{bodem},s1}, 1.5 h_{ef,s1} \Rechtsaf) \Rechtsaf)
\)
\(
B_{Nc} = links( \min links( 75 \, \tekst{mm}, 1.5 \keer 130 \, \tekst{mm} \Rechtsaf) \Rechtsaf) + \links( \min links( 475 \, \tekst{mm}, 1.5 \keer 130 \, \tekst{mm} \Rechtsaf) \Rechtsaf)
\)
\(
B_{Nc} = 270 \, \tekst{mm}
\)
De volgende, We evalueren de factored basic concrete breakout resistance van een enkel anker met behulp van CSA A23.3:19 Eq. D.6
\(
N_{br} = k_c \phi \lambda_a \sqrt{\frac{f'_c}{\tekst{MPa}}} \links( \frac{h_{ef,s1}}{\tekst{mm}} \Rechtsaf)^{1.5} R N
\)
\(
N_{br} = 10 \keer 0.65 \keer 1 \keer sqrt{\frac{20.68 \, \tekst{MPa}}{1 \, \tekst{MPa}}} \keer links( \frac{130 \, \tekst{mm}}{1 \, \tekst{mm}} \Rechtsaf)^{1.5} \keer 1 \keer 0.001 \, \tekst{kN} = 43.813 \, \tekst{kN}
\)
Waarbij,
- \(zodat ingenieurs precies kunnen nagaan hoe deze berekeningen zijn gemaakt{c} = 10\) voor ingestorte ankers
- \(\lambda = 1.0 \) for normal-weight concrete
Nu, we assess the effects of geometry by calculating the edge effect factor.
De kortste randafstand van de ankergroep wordt bepaald als:
\(
c_{een,\tekst{min}} = min links( c_{\tekst{links},s1}, c_{\tekst{Rechtsaf},s1}, c_{\tekst{top},s1}, c_{\tekst{bodem},s1} \Rechtsaf) = min links( 475 \, \tekst{mm}, 75 \, \tekst{mm}, 75 \, \tekst{mm}, 475 \, \tekst{mm} \Rechtsaf) = 75 \, \tekst{mm}
\)
Volgens CSA A23.3:19 Eq. D.10 and D.11, the breakout edge effect factor is:
\(
\Psi_{ed,N} = min links( 1.0, 0.7 + 0.3 \links( \frac{c_{een,\tekst{min}}}{1.5 h_{ef,s1}} \Rechtsaf) \Rechtsaf) = min links( 1, 0.7 + 0.3 \keer links( \frac{75 \, \tekst{mm}}{1.5 \keer 130 \, \tekst{mm}} \Rechtsaf) \Rechtsaf) = 0.81538
\)
Daarnaast, Beide cracking factor als de splitting factor worden genomen als:
\(
\Psi_{c,N} = 1
\)
\(
\Psi_{cp,N} = 1
\)
Vervolgens, we combine all these factors and use ACI 318-19 Eq. 17.6.2.1b om de factored concrete breakout resistance of the single anchor:
\(
N_{cbr} = links( \frac{EEN_{Nc}}{EEN_{Onthouden}} \Rechtsaf) \Psi_{ed,N} \Psi_{c,N} \Psi_{cp,N} N_{br} = links( \frac{72900 \, \tekst{mm}^ 2}{152100 \, \tekst{mm}^ 2} \Rechtsaf) \keer 0.81538 \keer 1 \keer 1 \keer 43.813 \, \tekst{kN} = 17.122 \, \tekst{kN}
\)
Bedenk de eerder berekende spanningsbelasting per anker:
\(
N_{fa} = frac{N_x}{N_{een,s}} = frac{50 \, \tekst{kN}}{4} = 12.5 \, \tekst{kN}
\)
Sinds 12.5 kN < 17.122 kN De betonnen breakout -capaciteit is voldoende.
This concrete breakout calculation is based on Anchor ID #1. The same capacity will apply to the other anchors due to the symmetric design.
Controleren #5: Bereken het pull -outcapaciteit van het anker
The pullout capacity of an anchor is governed by the resistance at its embedded end. For hooked anchors, it is dependent on its hook length.
We compute the factored basic anchor pullout resistance per CSA A23.3:19 Eq. D.17.
\(
N_{pr} = \Psi_{c,p} 0.9 \phi (f'_c) e_h d_a R = 1 \keer 0.9 \keer 0.65 \keer (20.68 \, \tekst{MPa}) \keer 60 \, \tekst{mm} \keer 19.05 \, \tekst{mm} \keer 1 = 13.828 \, \tekst{kN}
\)
Bedenk de eerder berekende spanningsbelasting per anker:
\(
N_{fa} = frac{N_x}{N_{een,t}} = frac{50 \, \tekst{kN}}{4} = 12.5 \, \tekst{kN}
\)
Sinds 12.5 kN < 13.828 kN, De pull -outcapaciteit van het anker is voldoende.
Controleren #6: Bereken de side-face blowoutcapaciteit in Y-richting
This calculation is not applicable for hooked anchors.
Controleren #7: Bereken side-face blowoutcapaciteit in Z-richting
This calculation is not applicable for hooked anchors.
Ontwerp Samenvatting
De Skyciv Base Plate Design Software Kan automatisch een stapsgewijze berekeningsrapport genereren voor dit ontwerpvoorbeeld. Het biedt ook een samenvatting van de uitgevoerde controles en hun resulterende verhoudingen, De informatie in één oogopslag gemakkelijk te begrijpen maken. Hieronder is een sample samenvattende tabel, die is opgenomen in het rapport.
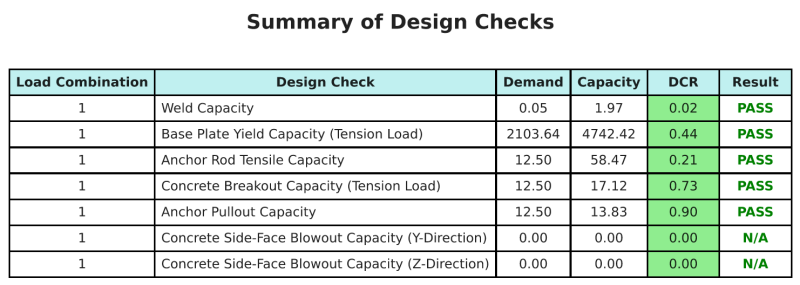
Skyciv Sample Report
Sample report will be added soon.
Koop baseplaatsoftware
Purchase the full version of the base plate design module onits own without any other SkyCiv modules. Dit geeft u een volledige set resultaten voor het ontwerp van de basisplaat, inclusief gedetailleerde rapporten en meer functionaliteit.