目录

• 了解垫子基础上的钢筋发育长度
• 压缩展开长度
• 张力发展长度
• SkyCiv 基础设计模块

该文档探讨了钢筋发展长度在具体基础上的重要性及其在确保结构完整性中的作用. 您可以深入了解设计代码要求, 影响发展长度的因素, 以及将它们纳入您的基础设计的实用方法. 加, 发现SkyCiv基础设计模块如何简化验证项目的钢筋开发长度的过程.

了解垫子基础上的钢筋发育长度

适当的锚固和增强对于混凝土结构的稳定性和寿命至关重要, 特别是在垫子的基础上. 开发长度是嵌入在钢和混凝土之间所需键强的混凝土中的钢筋的最小长度. 开发长度检查可确保加固充分嵌入以抵抗载荷而无需滑动, 保持结构完整性并使安全负载转移到地面. 验证发展长度是基础设计的关键部分, 确保在静态和动态载荷下的性能并保护整体结构稳定性.

不同的设计标准提供了确定这些长度的特定指南. 本文概述了根据各种设计标准指定的基础发展长度要求, 包括ACI 318-14 (美国混凝土研究所), 如 3600 (澳大利亚标准), CSA (加拿大标准协会), 和EN (欧洲规范). 通过检查每个标准规定的不同方法和标准, 工程师可以更好地了解如何在实践中有效应用这些准则, 确保坚固且兼容的结构设计.

压缩展开长度

基础的压缩发育长度是确定其所需厚度以确保加固条正确锚固的关键因素. 该长度是根据需要充分嵌入混凝土中的条杆以达到足够键强的需要计算的. 合并正确的开发长度使工程师可以设计具有最佳厚度的基础以进行加固, 确保结构稳定性和耐用性并提高整体安全性.

美国混凝土研究所 (ACI 318 部分 25.4.9)

公制:

\(由使用公式计算的最小值控制{直流} = max left[ \压裂{0.24 F_{和} \psi_{[R}}{\lambda sqrt{F'_{C}}} \d_{b}, 0.042 F_{和} \psi_{[R} d_{b}, 200MM 对]\)英制:

\(由使用公式计算的最小值控制{直流} = max left[ \压裂{F_{和} \psi_{[R}}{50 \λ sqrt{F'_{C}}} \d_{b}, 0.0003 F_{和} \psi_{[R} d_{b}, 8英寸右]\)在哪里:

F_和 =钢筋屈服强度 (兆帕, 压力)
F’_C =具体强度 (兆帕, 压力)
d_b =销杆直径 (毫米, 在)
ѱ _[R =限制强化因子 (桌子 25.4.9.3)
ƛ=混凝土类型因子 (桌子 25.4.9.3)

澳大利亚标准 (如 3600 部分 13.1.5)

基本发展长度:

\(由使用公式计算的最小值控制{他的,CB} = max left[ \压裂{0.22 F_{他的}}{ \sqrt{F_{C}’}} \d_{b}, 0.0435 F_{他的} d_{b}, 200MM 对]\)在哪里:

F_他的 =钢筋屈服强度 (兆帕)
F_C‘ =具体强度
d_b =启动杆直径 (毫米)

加拿大标准协会 (CSA部分 12.3)

\(由使用公式计算的最小值控制{D b} = max left[ \压裂{0.24 F_{和}}{ \sqrt{F_{C}’}} \d_{b}, 0.045 F_{和} d_{b}, 200MM 对]\)在哪里:

F_和 =钢筋屈服强度 (兆帕)
F_C‘ =具体强度
d_b =销杆直径 (毫米)

欧洲规范 (在节中 8.4)

基本的锚固长度 (8.4.3)

\(由使用公式计算的最小值控制{b,RQD} = frac{\φ}{4} \时代 frac{\sigma_{SD}}{F_{BD}} \)在哪里:

F_和 =钢筋屈服强度 (兆帕)
F_BD =最终键应力 (兆帕)
σ_SD =杆的设计应力，位于从锚定的位置测量的位置 (兆帕)
ɸ=销钉直径 (毫米)

设计锚固长度 (8.4.4)

\(由使用公式计算的最小值控制{BD} = alpha_{1} \α_{2} \α_{3} \α_{4} 由使用公式计算的最小值控制{b,RQD} \)在哪里:

一种₁, 一种₂, 一种₃, 一种₄ = 1.0 用于压缩 (桌子 8.2)

最小锚固长度 (8.4.4)

\(由使用公式计算的最小值控制{b, 分} = max left[ 0.6 由使用公式计算的最小值控制{b,RQD}, 10ɸ, 100MM 对]\)压缩长度

\(由使用公式计算的最小值控制{BD,压缩} = max left[ 由使用公式计算的最小值控制{b, 分}, 由使用公式计算的最小值控制{BD}\对]\)

张力发展长度

张力的发展长度是确保基础的尺寸足以固定抗压力的固定尺寸的关键. 这个长度, 计算以达到混凝土和钢筋之间必要的键强度, 直接影响基础的大小和设计. 正确确定张力的发展长度使工程师可以设计能够固定加固的基础, 使结构能够承受拉伸应力并保持稳定性和性能.

美国混凝土研究所 (ACI 318 部分 25.4)

直条纹 (部分 25.4.2.3)

公制:

\(由使用公式计算的最小值控制{d} = max left[ \剩下( \压裂{F_{和}}{1.1 \λ sqrt{F'_{C}}} \时代 frac{\psi_{!} \psi_{2} \PSI_3}{\剩下(C_{b} + K_{tr} \对) / d_{b}} \对)\d_{b}, 300MM 对]\)英制:

\(由使用公式计算的最小值控制{d} = max left[ \剩下( \压裂{3 F_{和}}{40\λ sqrt{F'_{C}}} \时代 frac{\psi_{!} \psi_{2} \PSI_3}{\剩下(C_{b} + K_{tr} \对) / d_{b}} \对) \d_{b}, 12在右]\)

在哪里:

ѱ_Ť =铸造位置因子 (桌子 25.4.2.4)
ѱ_Ë =棒涂料因子 (桌子 25.4.2.4)
ѱ_s =条尺寸因子 (桌子 25.4.2.4)
C_b =最小条形距离距离 (毫米, 在)
ķ_tr =横向加固指数 (毫米, 在)
(C_b + ķ_tr) / d_b ≤ 2.5

标准钩杆 (部分 25.4.3.1)

公制:

\(由使用公式计算的最小值控制{d} = max left[ \剩下( \压裂{0.24 F_{和} \psi_{Ë} \psi_{C} \psi_{[R}}{\λ sqrt{F'_{C}}} \对)\d_{b}, 8d_{b}, 150 MM 对]\)英制:

\(由使用公式计算的最小值控制{d} = max left[ \剩下( \压裂{F_{和} \psi_{Ë} \psi_{C} \psi_{[R}}{50 \λ sqrt{F'_{C}}} \对)\d_{b}, 8d_{b}, 6 在右]\)

在哪里:

ѱ_Ë =棒涂料因子 (桌子 25.4.3.2)
ѱ_C =吧台混凝土覆盖因子 (桌子 25.4.3.2)
ѱ_[R =限制加固因子 (桌子 25.4.3.2)

澳大利亚标准 (如 3600 部分 13.1.2.2)

基本发展长度:

\(由使用公式计算的最小值控制{他的,TB} = max left[ \压裂{0.5 钢底板设计欧洲规范{1} 钢底板设计欧洲规范{3} F_{和} d_{b}}{钢底板设计欧洲规范{2} \sqrt{F'_{C}}}, 0.058 F_{和} 钢底板设计欧洲规范{1} d_{b} \对]\)在哪里:

ķ₁ = 1.3 对于钢筋超过 300 MM混凝土铸件在杆下方 (1.0 否则)
ķ₂ = (132 – d_b)/100
ķ₃ = 1-[0.15(C_d – d_b)/d_b]
C_d =最小条形距离距离 (毫米)

直杆:

\(由使用公式计算的最小值控制{他的,Ť} = l_{他的,TB}\)

标准钩或齿轮:

\(由使用公式计算的最小值控制{他的,Ť} = 0.5 times l_{他的,TB}\)

加拿大标准协会 (CSA部分 12)

直条纹 (部分 12.2.3)

\(由使用公式计算的最小值控制{d} = max left[ 0.45 钢底板设计欧洲规范{1} 钢底板设计欧洲规范{2} 钢底板设计欧洲规范{3} 钢底板设计欧洲规范{4} \压裂{F_{和}}{\sqrt{F'_{C}}} d_{b}, 300 MM 对]\)在哪里:

ķ₁ =条位置因子 (12.2.4)
ķ₂ =涂料因子 (12.2.4)
ķ₃ =混凝土密度因子 (12.2.4)
ķ₄ =条尺寸因子 (12.2.4)

标准钩杆 (部分 12.5)

\(由使用公式计算的最小值控制{d} = max left[ \压裂{100 d_{b}}{\sqrt{F'_{C}}}\时代左(0.7 \压裂{F_{和}}{40}\对), 8 d_{b}, 150 MM 对]\)

欧洲规范 (在节中 8.4)

基本的锚固长度 (8.4.3)

\(由使用公式计算的最小值控制{b,RQD} = frac{\φ}{4} \时代 frac{\sigma_{SD}}{F_{BD}} \)设计锚固长度 (8.4.4)

\(由使用公式计算的最小值控制{BD} = alpha_{1} \α_{2} \α_{3} \α_{4} 由使用公式计算的最小值控制{b,RQD} \)在哪里:

一种₁, 一种₂, 一种₃, 一种₄ =表中显示的值 8.2 紧张的条

最小锚固长度 (8.4.4)

\(由使用公式计算的最小值控制{b, 分} = max left[ 0.3 由使用公式计算的最小值控制{b,RQD}, 10ɸ, 100MM 对]\)压缩长度

\(由使用公式计算的最小值控制{BD,紧张} = max left[ 由使用公式计算的最小值控制{b, 分}, 由使用公式计算的最小值控制{BD}\对]\)

有关SkyCiv设计模块如何验证开发长度的详细指南, 请参阅以下链接:

• 美国混凝土研究所 (ACI 318)
• 澳大利亚标准 (如 3600)
• 加拿大标准协会 (CSA A23.3)
• 欧洲规范 (在 1992)

SkyCiv 基础设计模块

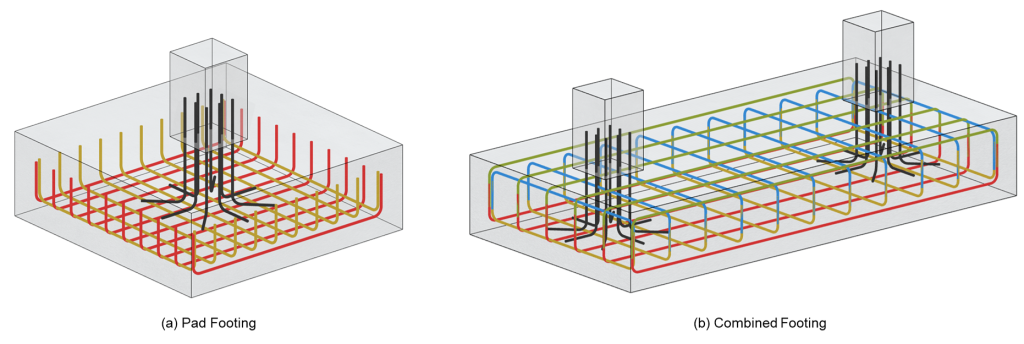
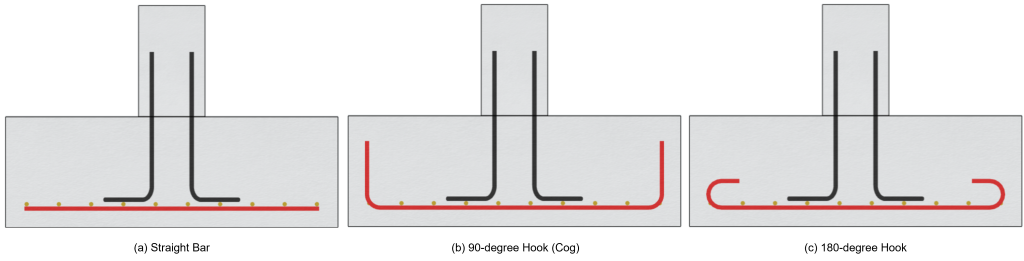
SkyCiv基金会设计模块的最新更新通过引入合并标准挂钩增援的能力来增强其功能, 实现更精确和详细的发展长度检查. 这项新功能通过允许用户自定义基础杆每一端的加固细节来为用户提供更大的灵活性. 用户现在可以指定加固作为直杆的结束, 90-学位钩 (齿轮), 或180度钩, 满足各种设计要求和标准.

该模块还具有更新的图形，可在视觉上有助于检查加固详细检查检查. 现在在3D图形中也可见列销或入门栏. 在其他标签下，新添加的求解器设置, 用户可以切换以忽略特定的设计检查, 例如开发长度检查和其他高级求解选项.

 

想要试用SkyCiv的Foundation Design软件? 我们的免费工具允许用户执行 混凝土基础计算 无需任何下载或安装!

免费的混凝土基础计算器