本文讨论两个钢筋混凝土板设计示例, 包括单向和双向弯曲. 主要目标是比较手工计算和 SkyCiv 板设计模块之间获得的结果. 我们将使用欧洲规范 2 用于钢筋混凝土结构.

建筑规范在定义板的典型案例时有类似的方法. 如果您想了解更多有关此主题的信息, 我们建议您阅读以下有关楼板设计的文章 ACI 平板设计示例和与 SkyCiv 的比较 和 澳大利亚标准 AS3600 楼板设计实例及与 SkyCiv 的比较

单向板设计示例

第一个要分析的案例是一座小型单层建筑 (数字 1, 数字 2) 其平板行为被描述为单向.

数字 1. 小型建筑中的单向板示例. (结构3D, SkyCiv 云工程).

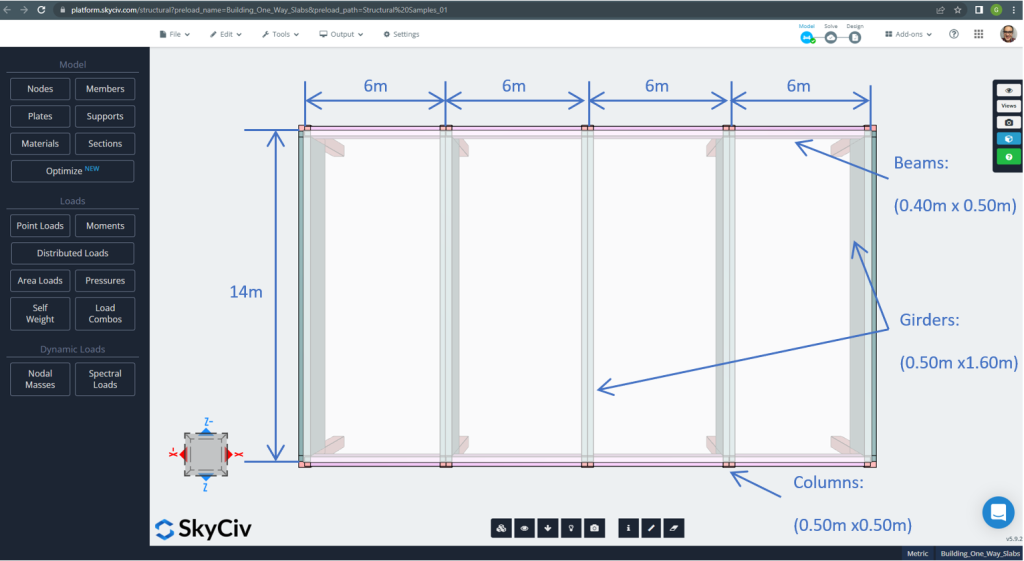
数字 2. 小型建筑中的单向板示例 (平面尺寸). (结构3D, SkyCiv 云工程).

对于平板示例, 总之, 材料, 元素属性, 和需要考虑的负载 :

• 板型分类: 一 – 方式行为 \(\压裂{L_2}{L_1} > 2 ; \压裂{14米}{6米}=2.33 > 2.00 \) 好的!
• 建筑占用: 住宅用途
• 板坯厚度 \(t_{平板}=0.25m)
• 钢筋混凝土密度 \(\罗_w = 25 \压裂{千牛}{米^3}\)
• 混凝土特性抗压强度 28 天 (C25\30) \(妈的= 25 兆帕 \)
• 板坯自重 \(死亡 = rho_w times t_{平板} = 25 \压裂{千牛}{米^3} \乘以 0.25m = 6.25 \压裂 {千牛}{米^2}\)
• 超载恒载 \(标准差= 3.0 \压裂 {千牛}{米^2}\)
• 活荷载 \(L = 2.0 \压裂 {千牛}{米^2}\)

根据 EN-2 进行手工计算

在这个部分, 我们将参考欧洲规范标准计算所需的钢筋. 我们首先获得由板的单一宽度条带执行的总因子弯矩.

• 恒载, \(g = (3.0 + 6.25) \压裂{千牛}{米^2} \次 1 m = 9.25 \压裂{千牛}{米}\)
• 活荷载, \(q = (2.0) \压裂{千牛}{米^2} \次 1 m = 2.0 \压裂{千牛}{米}\)
• 极限载荷, \(Fd = 1.35\times g + 1.5\乘以 q = (1.35\次 9.25 + 1.5\次 2.0)\压裂{千牛}{米} =15.5 \frac{千牛}{米} \)

获取钢筋面积前, 我们必须检查跨度有效深度比. 两个主要案例:

结构体系		基本跨有效深比
	结构系统系数 K	混凝土高应力 %(\(\罗 = 1.5 )\)	混凝土轻微受力 %(\(\罗 = 0.5 )\)
1. 连续梁或单向连续板或在一侧长边上连续的双向板的端跨	1.3	18	26
2. 连续梁或单向或双向跨板的内跨	1.5	20	30

最关键的情况是第一个情况, 所以, 我们选择一个比例 26.

• \(t_{分}= 分数{大号}{我知道}+cover+0.5\dot bar_{直径}= 分数{6米}{26}+0.025m+0.5\times 12mm=0.26m \) 〜 \(0.25米). 整体厚度还是足够的, 好的!

现在, 是时候使用该工作台来处理单向连续板了:

	结束支撑条件				首先是内部支撑	位于内部跨度的中间	在内部支撑处
	固定		连续
	外支撑	靠近端跨中部	结束支持	端跨
时刻	0	0.086FL	–	0.075FL	–	0.063FL	–
			0.04FL		0.086FL		0.063FL
剪力	0.4F	–		–		–
			0.46F		0.6F		0.5F

在哪里:

• L 是有效跨度
• F是跨度内的总极限荷载 (1.35Gk + 1.5Qk; Gk 是静载荷，Qk 是活载荷, 分别)

仅解释一种情况 (连续末端支撑) 其余部分如下表所示.

• \(F=Fd\times L = 15.5 \压裂{千牛}{米} \乘以 6m = 93.0 千牛 \)
• \(M=0.04FL=0.04 \times 93.0 kN \times 6m= -22.32{千牛}{米}\)
• \(d = 230 毫米 \)
• \(K=\frac{m}{{b}{d^2}{F_{钢底板设计欧洲规范}}}= 分数{22.32\乘以 10^6 {ñ}{毫米}}{{1000毫米}\次{(230 毫米)^ 2}\次 {25 \压裂{ñ}{毫米^2}}}=0.016877\)
• \(l_a = 0.95 \)
• \(z=l_a \times d = 0.95\times 230mm = 218.50 mm\)
• \(A_s = frac{m}{{0.87}{F_{yk}}{与}}= 分数{22.32\乘以 10^6 {ñ}{毫米}}{0.87\次 500 {ñ}{毫米^2} \次 {218.50毫米} = 234.83 毫米^2 }\)
• \(一个_{s,分}=0.0013{b}{d}=0.0013\times 1000mm \times 230 mm =299 mm^2\)
• \(一个_{圣}=最大(作为, 一个_{s,分}) = 最大值(234.83, 299) 毫米^2 = 299 毫米^2 \)

片刻	外部负左	外部积极	外部负权	内部负左	内部积极	内部负权
M值, 千牛·米	22.32	35.15	41.85	48.00	35.15	35.15
ķ	0.0168	0.0266	0.03164	0.0362	0.0266	0.0266
与, 毫米	218.50	218.50	218.50	218.50	218.50	218.50
\(作为, mm^2\)	234.83	369.815	440.31	505.011	369.815	369.815
\(一个_{s,分},mm^2\)	299.00	299.00	299.00	299.00	299.00	299.00
\(一个_{圣} {毫米^2}\)	299.00	369.815	440.31	505.011	369.815	369.815

下一步是使用 SkyCiv 中的板设计模块计算钢筋钢筋. 请, 继续阅读以下部分!.

如果你是 SkyCiv 的新手, 自行注册并测试软件!

注册 SkyCiv!

SkyCiv S3D 板设计模块结果

本节涉及获取钢筋面积，但仅使用软件, 的 板材设计模块. 以简洁的方式, 我们只会通过图像展示结果或重要信息.

在分析模型之前, 我们必须定义板网格尺寸. 一些参考资料 (2) 推荐的壳单元尺寸为 1/6 短跨度或 1/8 长跨度的, 其中较短的一个. 遵循这个值, 我们有 \(\压裂{L2}{6}= 分数{6米}{6} = 1 米 \) 要么 \(\压裂{L1}{8}= 分数{14米}{8}=1.75m \); 我们以 1m 作为最大推荐尺寸，并采用 0.50m 的网格尺寸.

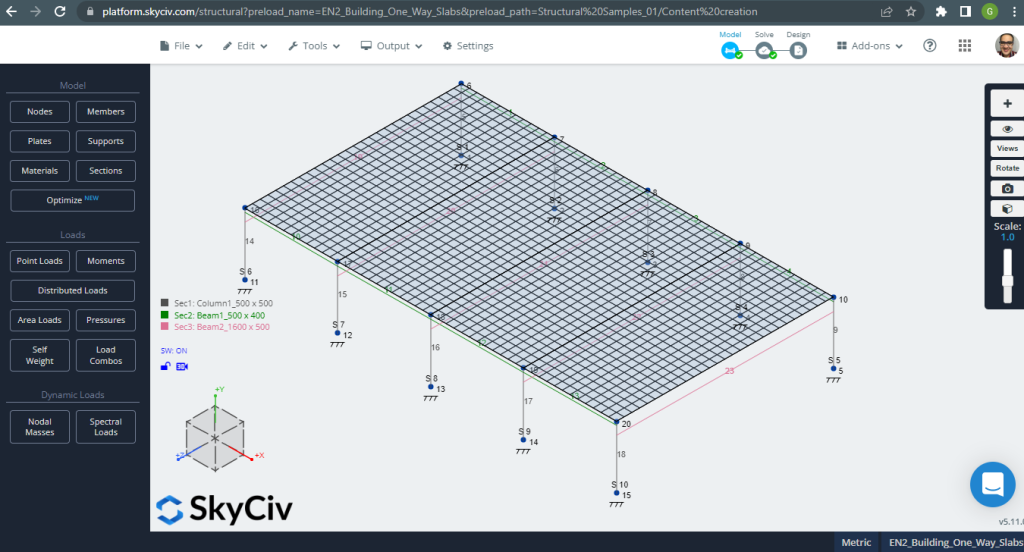
数字 3. 板网状. (结构3D, SkyCiv 云工程).

一旦我们改进了分析结构模型, 我们进行线性弹性分析. 设计楼板时, 我们必须检查垂直位移是否小于代码允许的最大值. Eurocode 2 建立了最大可维修性垂直位移 \(\压裂{大号}{250}= 分数{6000毫米}{250}=24.0 mm\).

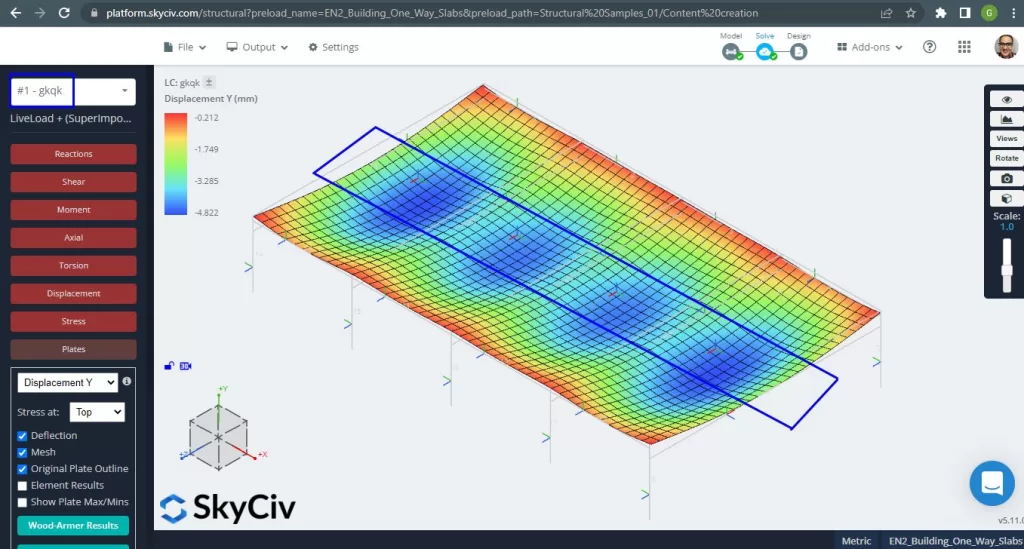
数字 4. 垂直位移, 跨度中心处的最大值. (结构3D, SkyCiv 云工程).

将最大垂直位移与规范参考值进行比较, 板的刚度足够. \(4.822 毫米 < 24.00mm\).

板跨度中的最大力矩位于中心，负力矩位于外部和内部支撑处. 让我们在下图中查看这些时刻值.

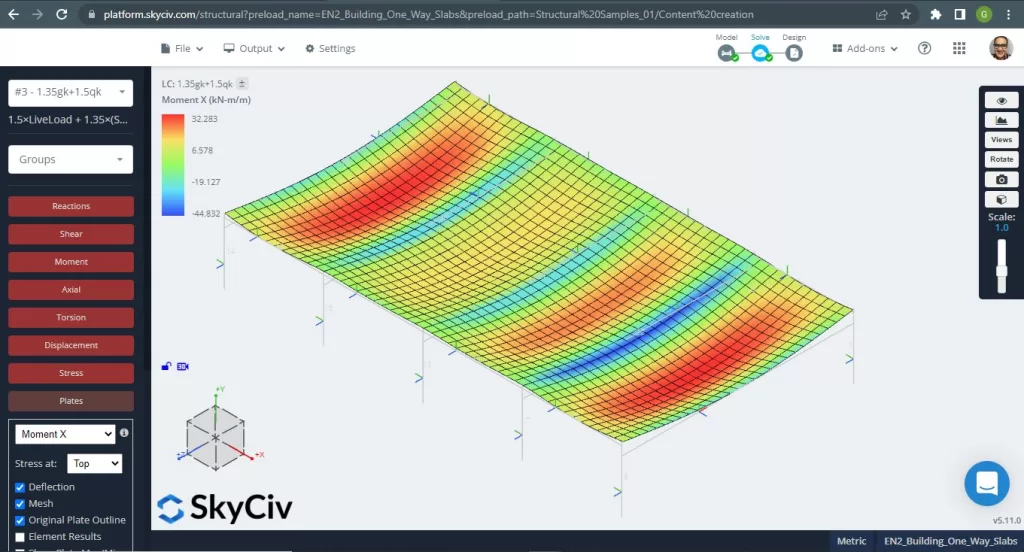
数字 5. X方向弯矩. (结构3D, SkyCiv 云工程).

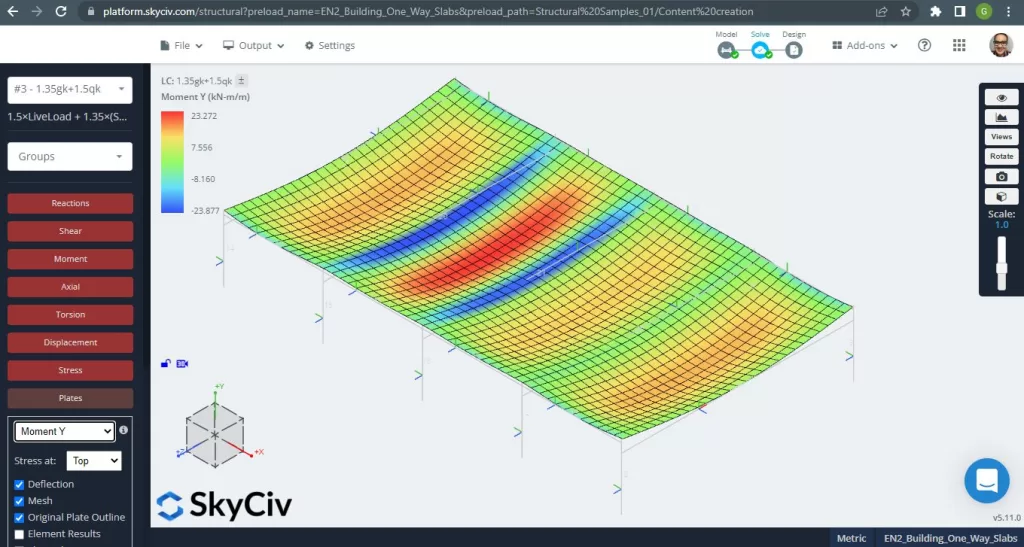
数字 6. Y方向弯矩. (结构3D, SkyCiv 云工程).

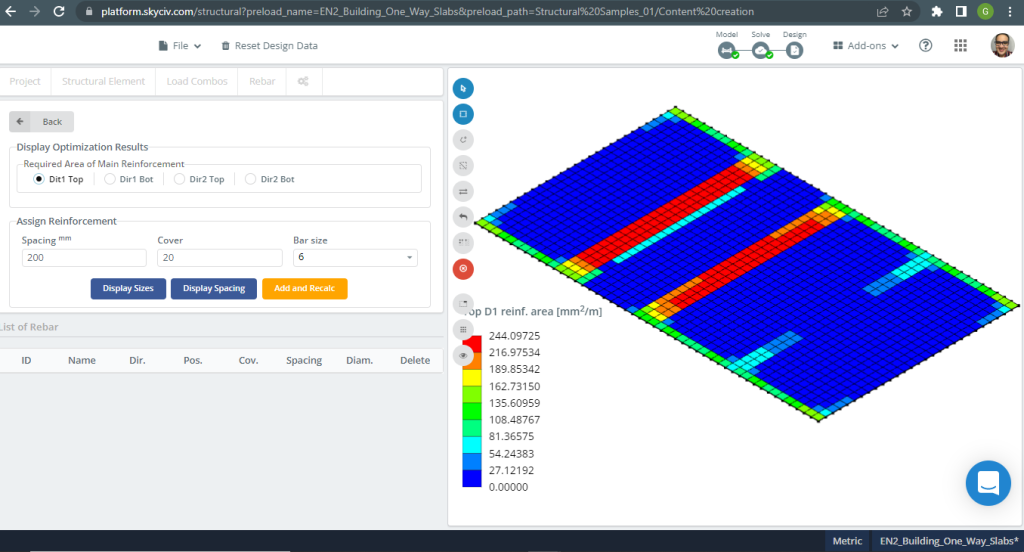
数字 7. 顶部 X 方向的钢筋. (结构3D, SkyCiv 云工程).

数字 8. 底部 X 方向钢筋. (结构3D, SkyCiv 云工程).

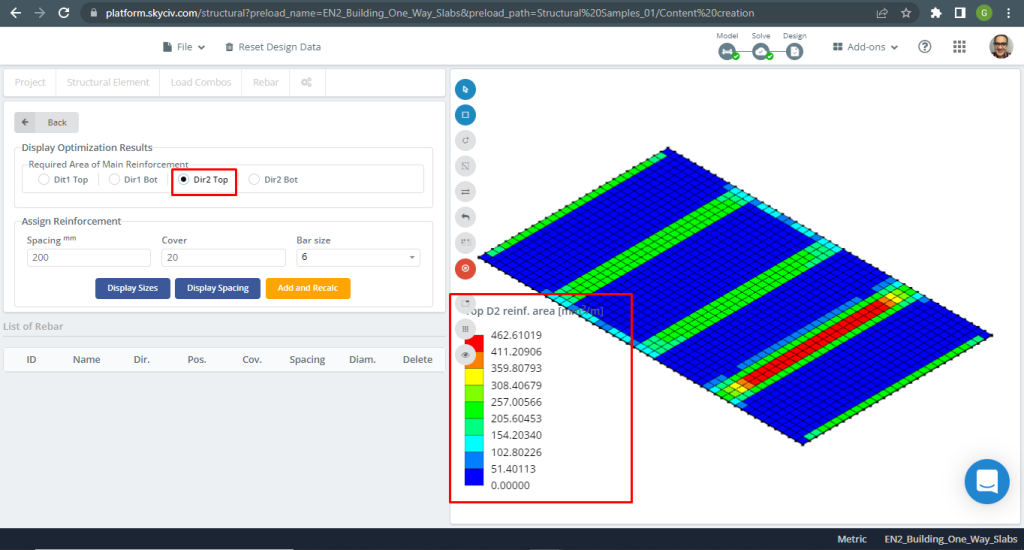
数字 9. 顶部 Y 方向钢筋. (结构3D, SkyCiv 云工程).

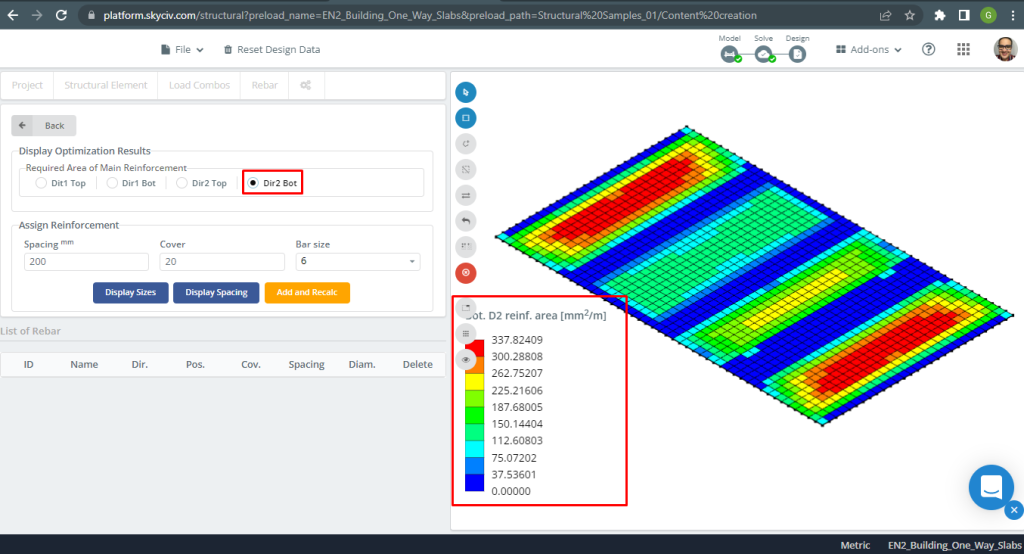
数字 10. 底部Y方向钢筋. (结构3D, SkyCiv 云工程).

结果比较

此单向板设计示例的最后一步是比较通过 S3D 分析获得的钢筋面积 (局部轴 “2”) 和手工计算.

力矩和钢面积	外部负左	外部积极	外部负权	内部负左	内部积极	内部负权
\(一个_{圣, 手算} {毫米^2}\)	299.00	369.82	440.31	505.011	369.82	369.82
\(一个_{圣, S3D} {毫米^2}\)	308.41	337.82	462.61	462.61	262.75	308.41
\(\三角洲_{差异}\) (%)	3.051	8.653	4.820	8.400	28.95	16.610

我们可以看到数值的结果非常接近. 这意味着计算是正确的!

如果你是 SkyCiv 的新手, 自行注册并测试软件!

注册 SkyCiv!

双向板设计示例

SkyCiv 3D 板设计模块是一款功能强大的软件，可以分析和设计您可以成像的任何类型的建筑. 对于第二个设计板示例, 我们决定运行平板系统 (数字 11).

数字 11. 小型建筑中的单向板示例. (结构3D, SkyCiv 云工程).

对于平板示例, 总之, 材料, 元素属性, 和需要考虑的负载 :

• 板型分类: 二 – 方式行为 \(\压裂{L_2}{L_1} \这 2 ; \压裂{7米}{6米}=1.17 \le 2.00 \) 好的!
• 建筑占用: 住宅用途
• 板坯厚度 \(t_{平板}=0.30m\)
• 钢筋混凝土密度 \(\罗_w = 25 \压裂{千牛}{米^3}\)
• 混凝土特性抗压强度 28 天 (C25\30) \(妈的= 25 兆帕 \)
• 板坯自重 \(死亡 = rho_w times t_{平板} = 25 \压裂{千牛}{米^3} \乘以 0.30m = 7.5 \压裂 {千牛}{米^2}\)
• 超载恒载 \(标准差= 3.0 \压裂 {千牛}{米^2}\)
• 活荷载 \(L = 2.0 \压裂 {千牛}{米^2}\)

根据 EN-2 进行手工计算

第一步是定义总极限载荷:

• 恒载, \(g = (3.0 + 7.5) \压裂{千牛}{米^2} \次 7 m = 73.50 \压裂{千牛}{米}\)
• 活荷载, \(q = (2.0) \压裂{千牛}{米^2} \次 7 m = 14.00 \压裂{千牛}{米}\)
• 极限载荷, \(Fd = 1.35\times g + 1.5\乘以 q = (1.35\次 73.50 + 1.5\次 14.00)\压裂{千牛}{米} =120.225 \frac{千牛}{米} \)

用于手工计算, 该结构必须分为一系列等效的框架. 我们可以通过以下方法来达到这个目标:

• 力矩分布 (哈迪十字法) 用于框架分析.
• 计算机框架分析的刚度方法. 试试我们的 刚度矩阵计算器.
• 一种使用单向力矩系数的简化方法，调整至以下要求 (我们选择这种方法是因为分析模型的简单性):
  • 横向稳定性不依赖于板柱连接 (我们不分析建筑物的横向荷载);
  • 在所考虑的方向上至少有三排跨度大致相等的面板 (我们在两个主要方向都有四排和三排面板);
  • 海湾尺寸超过 \(30m^2\) (我们的模型区域是 \(42m^2\)

为板示例选择的厚度大于下表中所示的耐火最大最小值.

标准耐火性	最小尺寸 (毫米)
	板坯厚度, HS	轴距, 一个
雷伊 60	180	15
雷伊 90	200	25
雷伊 120	200	35
雷伊 240	200	50

在这个部分, 我们将仅开发纵向和柱条的计算 (随意计算另一个方向, 横向的, 对于中间条带). 在深入研究数字之前, 首先我们要分成条状: 中间和柱子. (有关设计条的更多详细信息, 查看这篇 SkyCiv 文章: 使用 ACI-318 设计板).

• 柱条宽度: \(6m/4 = 1.50m\)
• 中带宽度: \(7米 – 2\times 1.50m = 4.0m\)

EC2 允许根据下表在每个设计条中分配力矩

	柱条	中条
边柱负力矩	100% 但不超过 \(0.17{是}{d^2}{F_{钢底板设计欧洲规范}}\)	0
内柱负力矩	60-80%	40-20%
跨度中的积极时刻	50-70%	50-30%

我们选择了正在分析的柱条的力矩百分比:

• 边柱负力矩: 100%.
• 内柱负力矩: 80%
• 跨度中的积极时刻: 70%

总设计带矩计算:

	结束支撑条件				首先是内部支撑	位于内部跨度的中间	在内部支撑处
	固定		连续
	外支撑	靠近端跨中部	结束支持	端跨
时刻	0	0.086FL	–	0.075FL	–	0.063FL	–
			0.04FL		0.086FL		0.063FL
剪力	0.4F	–		–		–
			0.46F		0.6F		0.5F

在哪里:

• L 是有效跨度
• F是跨度内的总极限荷载 (1.35Gk + 1.5Qk; Gk 是静载荷，Qk 是活载荷, 分别)

仅解释一种情况 (连续末端支撑) 其余部分如下表所示.

• \(F=Fd\times L = 120.225 \压裂{千牛}{米} \乘以 6m = 721.35 千牛 \)
• \(M=0.04FL=0.04 \times 721.35 kN \times 6m= -173.124 {千牛}{米}\)
• \(d = 280 毫米 \)
• \(K=\frac{m}{{b}{d^2}{F_{钢底板设计欧洲规范}}}= 分数{173.124\乘以 10^6 {ñ}{毫米}}{{1500毫米}\次{(280 毫米)^ 2}\次 {25 \压裂{ñ}{毫米^2}}}=0.012637\)
• \(l_a = 0.95 \)
• \(z=l_a \times d = 0.95\times 280mm = 266.0 mm\)
• \(A_s = frac{m}{{0.87}{F_{yk}}{与}}= 分数{173.124\乘以 10^6 {ñ}{毫米}}{0.87\次 500 {ñ}{毫米^2} \次 {266.0毫米} = 214.0523 毫米^2 }\)
• \(一个_{s,分}=0.0013{b}{d}=0.0013\times 1500mm \times 280 mm =546 mm^2\)
• \(一个_{圣}=最大(作为, 一个_{s,分}) = 最大值(234.83, 546) 毫米^2 = 299 毫米^2 \)

片刻	外部负左	外部积极	外部负权	内部负左	内部积极	内部负权
M值, 千牛·米	173.124	191.125	260.064	298.281	191.125	218.429
ķ	0.05897	0.06500	0.0884	0.101	0.06500	0.0743
与, 毫米	266.00	266.00	266.00	266.00	266.00	266.00
\(作为, mm^2\)	1498.366	1651.761	2247.55	2577.835	1651.761	1887.727
\(一个_{s,分},mm^2\)	546.00	546.00	546.00	546.00	546.00	546.00
\(一个_{圣} {毫米^2}\)	1498.366	1651.761	2247.55	2577.835	1651.761	1887.727

下一步是使用 SkyCiv 中的板设计模块计算钢筋钢筋. 请, 继续阅读以下部分!

SkyCiv S3D 板设计模块结果

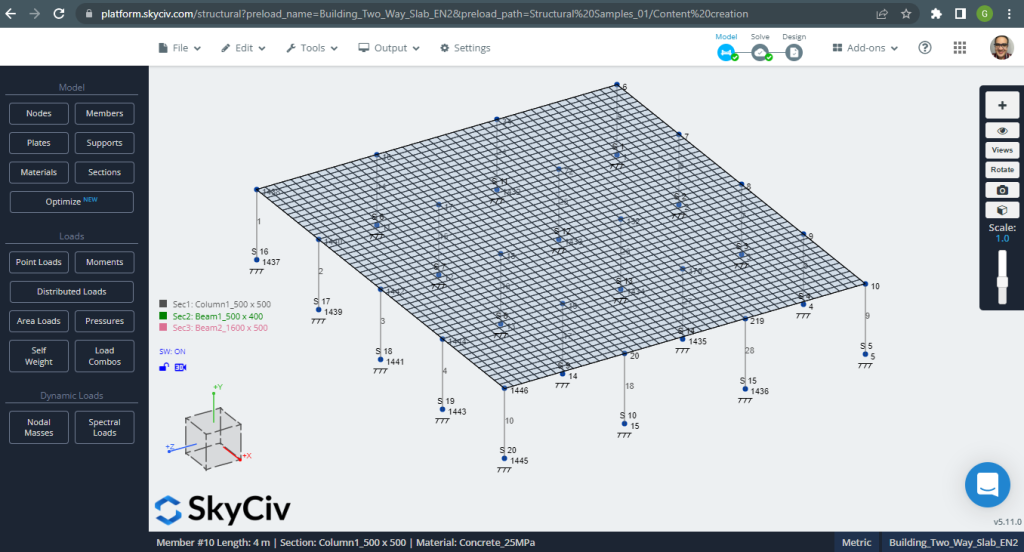
数字 12. 小型建筑中的单向板示例. (结构3D, SkyCiv 云工程).

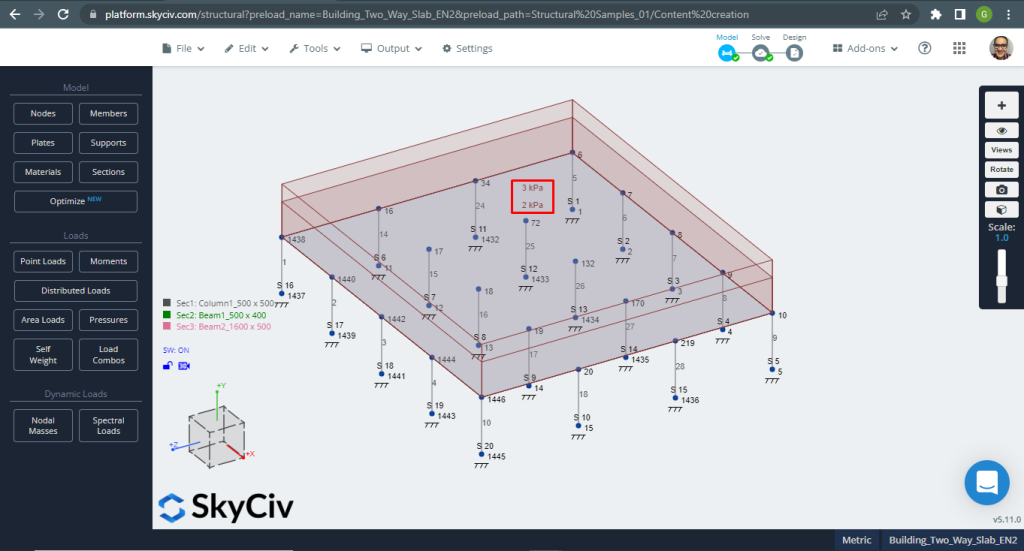
数字 13. 小型建筑中的单向板示例. (结构3D, SkyCiv 云工程).

设计楼板时, 我们必须检查垂直位移是否小于代码允许的最大值. 欧洲规范规定了最大可维修性垂直位移 \(\压裂{大号}{250}= 分数{6000毫米}{250}=24.0 mm\).

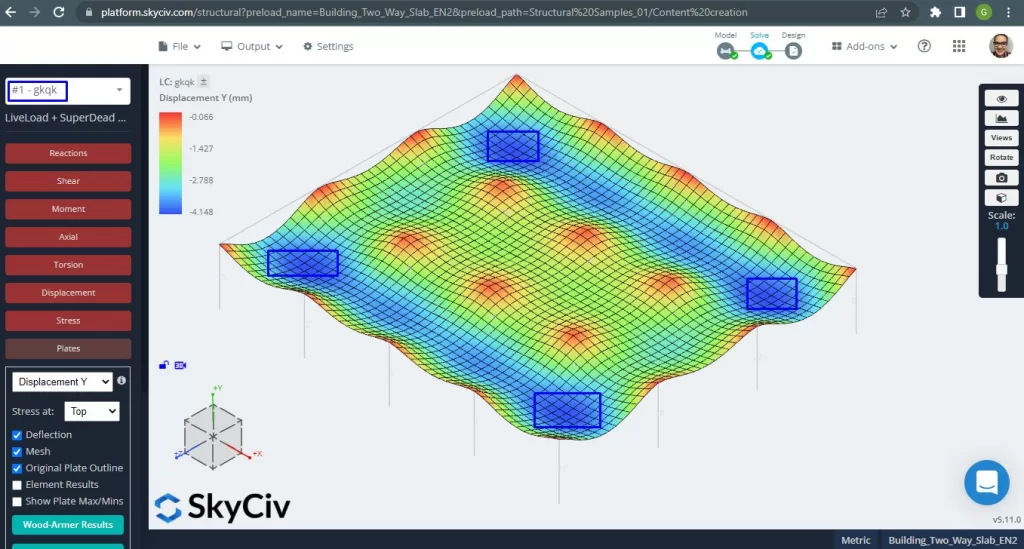
数字 14. 小型建筑中的单向板示例. (结构3D, SkyCiv 云工程).

上图为我们提供了垂直位移. 最大值为-4.148mm，小于允许的最大值-24mm. 因此, 板坯的刚度足够.

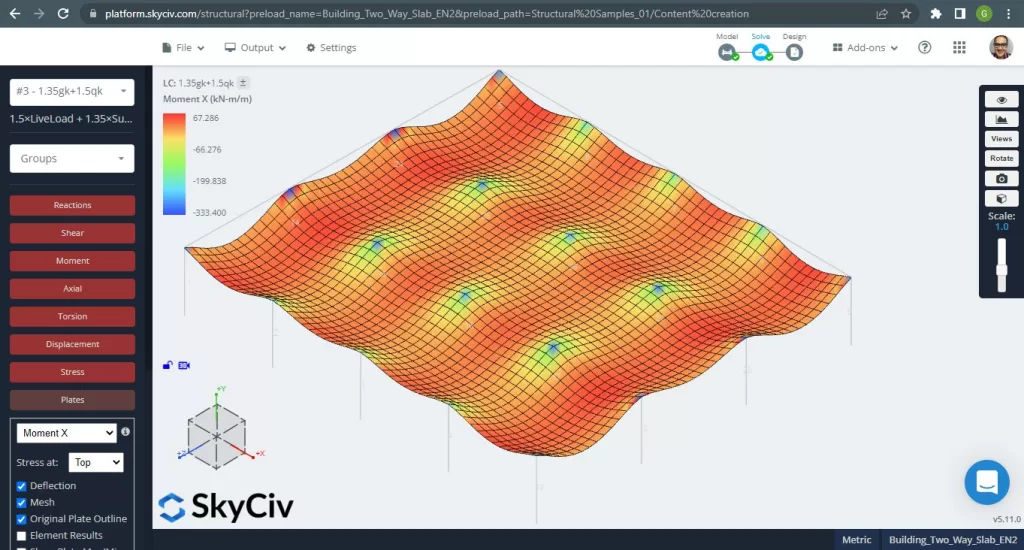
数字 15. 小型建筑中的单向板示例. (结构3D, SkyCiv 云工程).

图片 15 和 16 由每个主方向的弯矩组成. 获取矩分布和值, 软件, SkyCiv, 即可求得总钢筋面积.

数字 16. 小型建筑中的单向板示例. (结构3D, SkyCiv 云工程).

钢筋加固区域:

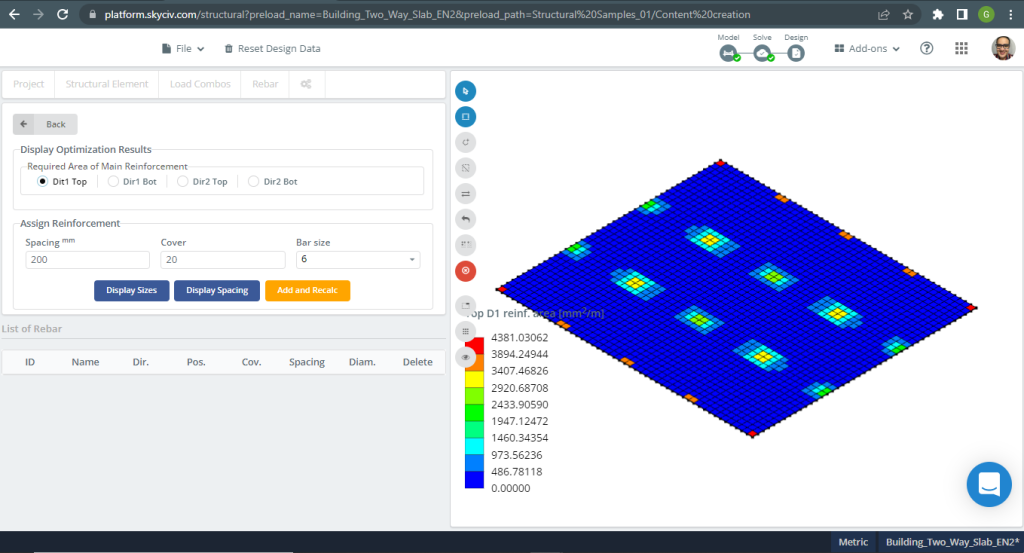
数字 17. 小型建筑中的单向板示例. (结构3D, SkyCiv 云工程).

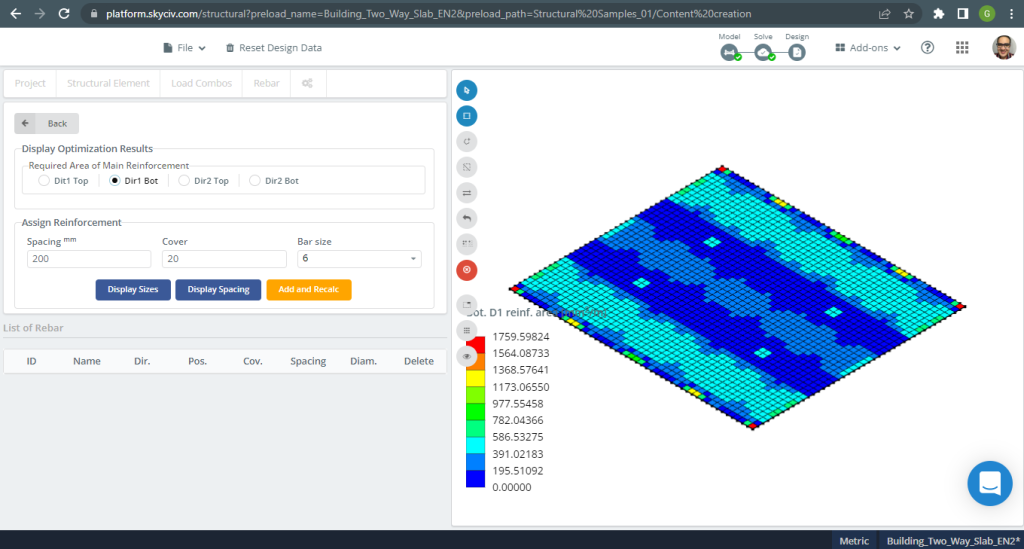
数字 18. 小型建筑中的单向板示例. (结构3D, SkyCiv 云工程).

数字 19. 小型建筑中的单向板示例. (结构3D, SkyCiv 云工程).

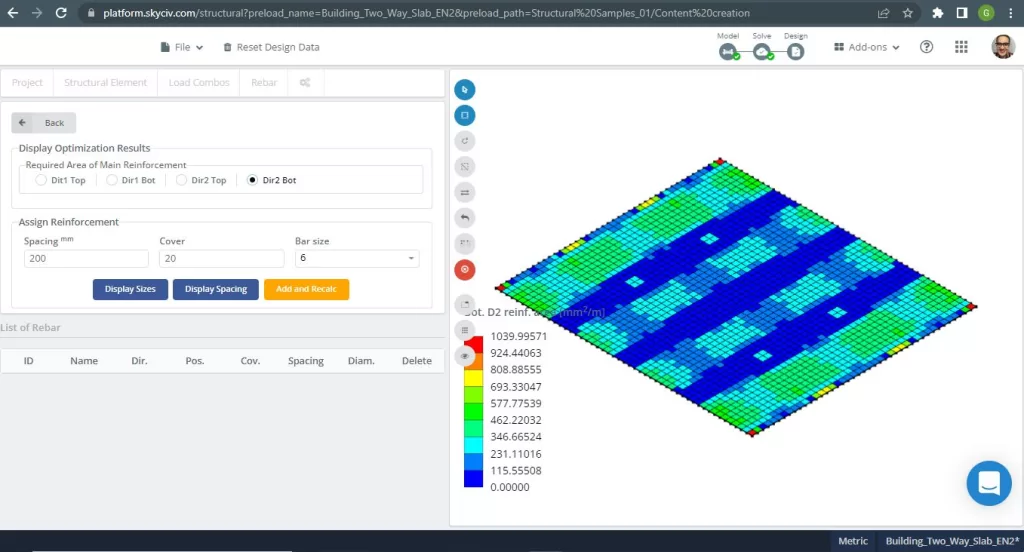
数字 20. 小型建筑中的单向板示例. (结构3D, SkyCiv 云工程).

结果比较

此双向板设计示例的最后一步是比较通过 S3D 分析和手动计算获得的钢筋面积.

X向钢筋及柱条

力矩和钢面积	外部负左	外部积极	外部负权	内部负左	内部积极	内部负权
\(一个_{圣, 手算} {毫米^2}\)	1498.366	1651.761	2247.55	2577.835	1651.761	1887.727
\(一个_{圣, S3D} {毫米^2}\)	3889.375	1040.00	4196.145	4196.145	520.00	3175.00
\(\三角洲_{差异}\) (%)	61.475	37.04	46.44	38.566	68.52	40.544

 

如果你是 SkyCiv 的新手, 自行注册并测试软件!

注册 SkyCiv!

参考资料

1. 乙. 莫斯利, [R. 赫尔斯, 杰赫. 蹦极 , “符合欧洲规范 2 的钢筋混凝土设计”, 第七版, 帕尔格雷夫·麦克米伦.
2. 巴赞·恩里克 & 梅利·皮拉拉, “结构抗震设计”, 1编辑, 清除.
3. Eurocode 2: 混凝土结构设计.