Пример дизайна базовой плиты с использованием en 1993-1-8-2005, В 1993-1-1-2005 и EN 1992-1-1-2004
Запись о проблеме
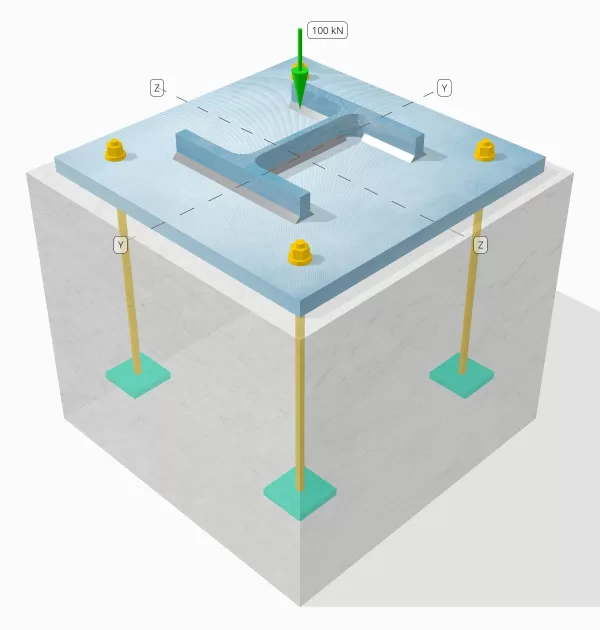
Определите, достаточным ли разработанное соединение с столбцом-базой для нагрузки с компрессионной нагрузкой на 100 кН..
Данные данных
Столбец:
Раздел столбца: ОН 200 В
Область столбца: 7808 мм2
Материал столбца: S235
Опорная плита:
Размеры опорной плиты: 400 мм х 400 мм
Толщина опорной плиты: 20 мм
Материал опорной плиты: S235
Раствор:
Толщина затирки: 20 мм
бетон:
Бетонные размеры: 450 мм х 450 мм
Бетонная толщина: 380 мм
Бетонный материал: C20/25
Швы:
Нагрузка сжатия переносится только через сварные швы? НЕТ
Model in SkyCiv Free Tool
Model the base plate design above using our free online tool today! No sign-up required.
Пошаговые расчеты
Проверьте #1: Рассчитайте емкость сварки
Поскольку нагрузка сжатия не переносится только через сварные швы, Надлежащая поверхность контактного подшипника требуется, чтобы гарантировать, что нагрузка переносится через подшипник. Обратиться к В 1090-2:2018 Пункт 6.8 для подготовки к контакту.
Дополнительно, use minimum weld size specified in Eurocode.
Проверьте #2: Calculate concrete bearing capacity and base plate yield capacity
The first step is to determine the design compressive strength of the joint, which depends on the geometry of the support (бетон) and the geometry of the loaded area (base plate).
We begin by calculating the alpha factor, which accounts for the diffusion of the concentrated force within the foundation.
В соответствии с В 1992-1-1:2004, Пункт 6.7, the alpha coefficient is the ratio of the loaded area to the maximum distribution area, which has a similar shape to the loaded area.
We will use the equation from Часть 6.1 of Multi-Storey Steel Buildings Part 5 по Arcelor Mittal, Peiner Träger, и Corus to calculate the alpha factor.
\(
\alpha = \min \left(
1 + \гидроразрыва{т_{\текст{концентрация}}}{\Максимум(L_{\текст{бп}}, B_{\текст{бп}})},
1 + 2 \осталось( \гидроразрыва{e_h}{L_{\текст{бп}}} \право),
1 + 2 \осталось( \гидроразрыва{e_b}{B_{\текст{бп}}} \право),
3
\право)
\)
\(
\alpha = \min \left(
1 + \гидроразрыва{380 \, \текст{мм}}{\Максимум(400 \, \текст{мм}, 400 \, \текст{мм})},
1 + 2 \осталось( \гидроразрыва{25 \, \текст{мм}}{400 \, \текст{мм}} \право),
1 + 2 \осталось( \гидроразрыва{25 \, \текст{мм}}{400 \, \текст{мм}} \право),
3
\право)
\)
\(
\альфа = 1.125
\)
где,
\(
e_h = \frac{L_{\текст{концентрация}} – L_{\текст{бп}}}{2} = frac{450 \, \текст{мм} – 400 \, \текст{мм}}{2} знак равно 25 \, \текст{мм}
\)
\(
e_b = \frac{B_{\текст{концентрация}} – B_{\текст{бп}}}{2} = frac{450 \, \текст{мм} – 400 \, \текст{мм}}{2} знак равно 25 \, \текст{мм}
\)
Once the geometry is defined, we will then determine the compressive strength of the concrete using В 1992-1-1:2004, уравнение. 3.15.
\(
f_{CD} = frac{\альфа_{cc} f_{ск}}{\Gamma_c} = frac{1 \раз 20 \, \текст{МПа}}{1.5} знак равно 13.333 \, \текст{МПа}
\)
следующий, we assume a value for the beta coefficient. Since grout is present, beta value can be 2/3. We will calculate the design bearing strength of the joint using the combined formulas from В 1993-1-8:2005 уравнение. 6.6, и В 1992-1-1:2004 уравнение. 6.63.
\(
f_{Джейд} = \beta \alpha f_{CD} знак равно 0.66667 \раз 1.125 \раз 13.333 \, \текст{МПа} знак равно 10 \, \текст{МПа}
\)
The second part involves calculating the base plate yield capacity.
Since we already have the design bearing strength of the connection, we will use this to determine the smallest cantilever distance of the base plate that experiences the full bearing load. We will refer to the SCI P358 example on page 243 и В 1993-1-1:2005 Пункт 6.2.5.
\(
c = t_{\текст{бп}} \SQRT{\гидроразрыва{f_{y_{\текст{бп}}}}{3 f_{Джейд} \гамма_{М0}}} знак равно 20 \, \текст{мм} \раз sqrt{\гидроразрыва{225 \, \текст{МПа}}{3 \раз 10 \, \текст{МПа} \раз 1}} знак равно 54.772 \, \текст{мм}
\)
We will use this dimension to calculate the effective area of the base plate. The ‘c’ dimension we calculated may overlap or not overlap near the flange. If it overlaps, we will assume the section to be a rectangular section. If it does not overlap, we will take the shape of the column.
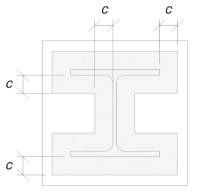
Without overlap
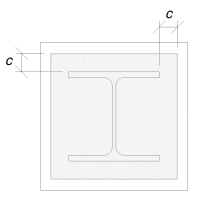
With overlap
We determined that the ‘c’ dimension does not overlap. Следовательно, с использованием SCI P358 pg. 243, the effective area is:
\(
A_e = 4c^2 + П_{\текст{полковник}}с + A_{\текст{полковник}} знак равно 4 \times 54.772^2 \, \текст{мм}^ 2 + 1182 \, \текст{мм} \раз 54.772 \, \текст{мм} + 7808 \, \текст{мм}^ 2 = 84549 \, \текст{мм}^ 2
\)
It is important to note that the effective area should not be less than the base plate area.
В завершение, мы будем использовать В 1993-1-8:2005 уравнение. 6.6, и EN 1992-1-1:2004, уравнение. 6.63 to calculate the design bearing resistance of the base plate connection.
\(
N_{Rd} = слева( \мин(А_е, A_0) \право) f_{Джейд} = слева( \мин(84549 \, \текст{мм}^ 2, 160000 \, \текст{мм}^ 2) \право) \раз 10 \, \текст{МПа} знак равно 845.49 \, \текст{кН}
\)
поскольку 845.49 кН > 100 кН, the design is достаточный!
Резюме дизайна
Программное обеспечение для проектирования базовых плитов Skyciv может автоматически генерировать пошаговый отчет расчета для этого примера проекта. Это также предоставляет краткую информацию о выполненных чеках и их полученных соотношениях, Облегчение информации для понимания с первого взгляда. Ниже приведена примерная сводная таблица, который включен в отчет.

Образец Skyciv
See the level of detail and clarity you can expect from a SkyCiv Base Plate Design Report. The report includes all key design checks, уравнения, and results presented in a clear and easy-to-read format. It is fully compliant with design standards. Click below to view a sample report generated using the SkyCiv Base Plate Calculator.
Покупка программного обеспечения для базовой пластины
Купите полную версию модуля дизайна базовой плиты без каких -либо других модулей Skyciv. Это дает вам полный набор результатов для дизайна базовой плиты, в том числе подробные отчеты и больше функциональности.


